English

• 1. INTRODUCTION

  In the semiconductor industry, germanium-based low-dimensional nanomaterials with high performance are gradually becoming potential candidates for non-silicon transistors^{[1, 2]}. How to develop new materials with high-performance properties has been the primary challenge for both theoretical and experimental scientists over the last decades. It has been widely used in a variety of crucial high-tech fields, for example, using germanium nanoparticles as a light-absorbing layer for solar cell^{[3, 4]}, as doping center of luminescent material application to LED device^{[5, 6]}, as a high performance broadband photo detector because of having excellent band gap^[7], and playing an important role in solid state batteries^[8].

  Binary germanium cluster, a class of excellent self-assembled nanomaterials, doped by transition metal (TM) or rare earth (RE) metal, have unique salient properties in the field of structural, electronic, magnetic, and optical aspects. As a suitable building block, stability is an essential factor determined by both geometric configurations and electronic structure^{[9, 10]}. In the early study of transition metal doped germanium cluster by Kumar and Kawazoe, the researchers performed DFT calculation to explore series of M@Ge_n (n = 14~16; M = Ti, Zr, Hf, Fe, Ru, Os) cluster and further comparatively analyzed the different growth behavior between M@Ge_n and M@Si_n including several possible cage structures of Frank-Kasper, fullerene-like, capped decahedral and cubic model^{[11, 12]}. From here on, transition metal doped germanium cluster with various elements has been studied^[13-20]. On another side of rare earth doped germanium cluster, Tang with his coworker studied the ground state geometry, energy gap, and optical gap of Ge₁₂M (M = Sc~Ni) by using density functional theory, and concluded that Ge₁₂M clusters having magnetic moments from 1 to 5 μ_B were regarded as potential magnetic materials with tunable magnetic properties^[21]. Borshch's group using density func-tional theory took the examination of ScGe_n^- (n = 6~16) cluster for optimization of spatial structure and electronic spectra comparably studied with experimental results^[22]. Qin et al. combine genetic algorithm with first-principles calculation aiming to find out the lowest-energy structure of Ge_nM^+/0 (n = 9, 10; M = Si, Li, Mg, Al, Fe, Mn, Pb, Au, Ag, Yb, Pm, and Dy)^[23]. In 2012, Nakajima's group utilized photoelectron spectroscopy to investigate a series of MGe_n (M = Sc, Ti, V, Y, Zr, Nb, Lu, Hf, and Ta). The results pointed that Ge₁₆ cage with larger cavity to encapsulate RE and TM atom formed anionic core shell structure, for which electronic and geometric closings are uniformly satisfied^[24]. Thereby, the RE and TM based germanium nanomaterials possess a number of great potential applications for next generation devices. That's why it is necessary to further study comprehensively.

  In this article, on the basis of Nakajima's previous experimental data^[24], we performed the growth behavior of LuGe_n^(+/0/-) (n = 6~19) and simulated photoelectron spectroscopy of LuGe_n^- (n = 6~19) cluster, focusing on the electronic structure of anionic LuGe₁₆^- as super atom with Frank-Kasper stable motif^[25]. Another way to say is that the goal of this research is to present the theoretical investigation of the effect of Lu (lutetium) metal doping on germanium clusters, while taking predicted optical and electronic properties into account for potential application to optoelectronic materials.

  2. COMPUTATIONAL DETAILS

  The initial structures are originated from: (1) By using the ABCluster unbiased global search technique, which can be seen as a random generator^{[26, 27]}. When it was combined with Gaussian software package, more than 400 initial guessed geometries for each LuGe_n^(+/0/-) (n = 6~19) were generated and optimized with the TPSSh functional combined with 6-31G for Ge atom and ECP60WMB for Lu (lutetium) atom by Gaussian 09 software package^{[28, 29]}. The energy change threshold as convergence condition is set to 10^-6 hartree. (2) The substitutional structure schemes were adopted, in which a Lu atom was substituted for a Ge in the most stable structure of Ge_n+1 cluster. Subsequently, the low-lying isomers are further optimized by DFT-TPSSh functional with all-electron cc-pVTZ basis set for Ge atom and ECP28MWB basis set for Lu^{[30, 31]}. Vibrational frequency analyses were performed to confirm those isomers are located at true minima on the potential energy surface. The single point energy calculations of the selected isomers were conducted by DFT-TPSSh functional with aug-cc-pVTZ basis set for Ge and ECP28MWB basis set for Lu. The PES spectra of LuGe_n^- (n = 6~19) were calculated by means of Koopmans' theorem^{[32, 33]}. The density of states (DOS) and partial density of states (PDOS) of LuGe₁₆^- have been obtained by Vienna Ab initio Simulation Package (VASP)^[34-37], with Perdew-Burke-Ernzerhof generalized gradient approximation (PBE-GGA) functional^[38]. The projector augmented wave (PAW) was set to explore the inert core electron^{[39, 40]}. To prevent interaction between adjacent clusters, the 40 × 40 × 40 Å edge lengths cubic cells with periodic boundary condition were taken into account. The plane wave cut-off energy was set up to 500 eV. The structures, isosurface maps, PES spectra and orbitals were created by visualization software of VMD and Multiwfn^{[41, 42]}.

  3. RESULTS AND DISCUSSION

  3.1 Ground state structure of LuGe_n^(+/0/-) (n = 6~19)

  The anionic, neutral and cationic clusters with stable geometries and point group are shown in Figs. 1~3, and the corresponding low-lying isomers with different energies are added in Figs. S1~S3, respectively. For anionic clusters, their ground states are predicted to be a singlet. For n = 6, the ground state structure is a pentagonal bipyramid with C_s symmetry which is the same as pure Ge₇ ground state structure where Lu atom replaces the top vertex of pyramid of the Ge atom^[43]. For n = 7, the ground state structure is a bi-capped tetragonal bipyramid with C_2v symmetry where two capped Ge atoms symmetrically adsorbed on its two faces. For n = 8, both 8a1 and 8a2 isomers compete for the ground state structure with each other owing to the fact that both of them are degenerated in energy (the energy difference is only 0.02 eV). 8a1 and 8a2 are both bi-capped pentagonal bipyramids, and the difference lies in the two capped Ge atom adsorption sites. The 9a geometry with C_s symmetry is based on 8a2 structure by adding a Ge atom. For n = 10~12, the 10a is a link structure where Lu atom works as a linker to link two orthogonal Ge₅ trigonal bipyramid (TBP), that for 11a is adding one Ge at 10a one side trigonal bipyramid to form two paralleled TBP and one Ge capped TBP, that for 12a is adding one Ge atom on each bilateral side of 10a. For n = 13, the Lu atom is located in the half cage center. The 13a1 and 13a2 are degenerated due to close energy difference of 0.04 eV. The 13a2 is hexagonal antiprism with C_6v symmetry where adsorbed one Ge atom on the hexagon surface and the 13a1 can be viewed that one Ge vertex of hexagonal antiprism of 13a2 moves to the triangular surface. For n = 14, the 14a is seen as adding one Ge atom on 13a2 with slight distortion. For n = 15~17, all 15a, 16a and 17a structures are derived from 16a of Frank-Kasper structure with T_d high-symmetry where the Lu atom locates at the cage center. The 15a is viewed as 16a by reducing one Ge atom from the bottom triangle and 17a is also viewed as 16a by adding one Ge atom on the bottom triangle. Both 15a and 17a have some extended distortion. For n = 18~19, these are all cage structures with Lu atom embedded in the center. 18a comes from the cage skeleton of 16a by adding two Ge atoms, with one in the middle layer and the other in the bottom. 19a consists of a six-membered ring symmetrical on both sides where each face capped one Ge atom, and a five-membered ring is at the middle symmetry plane to form an endohedral structure.

  Figure 1

  

  Figure 1.  The lowest-energy structures of LuGe_n^- (n = 6~19) with point group. The blue and red balls represent the Ge and Lu atoms, respectively

  DownLoad: Full-Size Img PowerPoint

  Figure 2

  

  Figure 2.  The lowest-energy structures of LuGe_n (n = 6~19) with point group. The blue and red balls represent the Ge and Lu atoms, respectively

  DownLoad: Full-Size Img PowerPoint

  Figure 3

  

  Figure 3.  The lowest-energy structures of LuGe_n⁺ (n = 6~19) with point group. The blue and red balls represent the Ge and Lu atoms, respectively

  DownLoad: Full-Size Img PowerPoint

  For neutral clusters, their ground states are calculated to be a doublet. For n = 6, it is the same as that anionic structure of pentagonal bipyramid. The difference is on the Lu atom that replaces the Ge atom site. For n = 7 and 8, there are one and two Ge atom capped LuGe₅ pentagonal bi-pyramids, respectively. For n = 9, its structure is equal to the pure Ge₁₀ cluster in which the Lu atom replaces a Ge atom on the top site. For n = 10 and 11, the 10n is a tetra-capped pentagonal bipyramid and the 11n is on that structure to add one Ge atom. For n = 12, the 12n is regarded as two sub-clusters of capped trigonal bipyramid linked by Lu atom. For n = 13 and 14, both are link-structure with Lu as the linker. In such structures, one side is the same as capped tetragonal antiprism and another side is four Ge atoms forming a trigonal pyramid for 13n. Five Ge atoms form a trigonal bipyramid for 14n. For n = 15, the 15n resembles to the 18n structure by removing Ge capped on each side at the ring face and one Ge atom on the symmetry plane. For n = 16, the 16n is distorted Frank-Kasper, and for n = 17 and 19, such structures are the same as that of the anionic one discussed above. For n = 18, the 18n cage skeleton is consistent with two five-membered rings as mirror symmetry where two Ge atoms are capped on each side at the ring face and the remaining four Ge atoms, in order to form rhombus, are coplanar on the symmetry plane.

  For cationic clusters, their ground states are evaluated to be singlet. For n = 6 and 7, the 6c is like 6n. And 7c is on that structure by adding one Ge atom. For n = 8 and 9, the 8c is a three Ge atom capped tetragonal bipyramid and 9c is based on 8c for more than one Ge atom. For n = 10, 10c is the same as the Ge₁₁ pure cluster^[44]. For n = 11~15, all of them are seen as link structures where Lu atom serves as the linker. 11c is two Ge₅ trigonal bipyramids which have jointly shared one Ge atom that is coplanar with Lu linker at the plane of symmetry. 12c is divided into one part of trigonal pyramid (Ge₃) and another part of tetragonal antiprism (Ge₈) linked by Lu at the center. 13c is based on 12c by adding one Ge atom at the tetragonal antiprism top site and 14c is based on 13c with the addition of one Ge atom at the trigonal pyramid site. 15c is two capped tetragonal antiprisms which share three Ge atoms on the central symmetry plane and Lu atom is on the center of that geometry. For n = 16 and 17, they are analogous to those of the corresponding neutral. For n = 18 and 19, 18c is built as Lu of center atom around two symmetrical six-membered rings and five-membered rings, with the remaining Ge atoms situated at three vertices of the triangle. 19c is the geometry for further epitaxial growth of 18c.

  In summary, from the above description, it can be concluded that: (1) The LuGe_n^(+/0/-) (n = 6~19) growth to be cage structure are clearly exhibited when n = 16. (2) The anion growth system has a distinct half cage phase. However, the cation and neutral cluster growth are not. (3) The energy surface of LuGe_n^(+/0/-) is so flat, which indicates distinguishing ground state geometries in the experiment needs more attention.

  3.2 PES spectra of LuGe_n^- (n = 6~19)

  The photoelectron spectra of different ground state isomers of LuGe_n^- (n = 6~19) determined theoretically and compared with the experimental spectra are shown in Fig. 4. For LuGe₆^-, the four peaks (x, a~c) are located at 2.90, 3.31, 3.98 and 4.84 eV which are highly consistent with experimental ones (X, A~C) at 2.95, 3.33, 4.02 and 4.82 eV. For LuGe₇^-, the five peaks (x, a~d) are placed at 2.72, 3.15, 3.77, 4.31 and 4.53 eV, in good agreement with the experimental data (X, A~D) of 2.70, 2.98, 3.45, 4.23 and 4.53 eV. For LuGe₈^-, two isomers of 8a1 and 8a2 have similar energy whose photoelectron spectra are shown. For 8a1, the six main peaks (x, a~e) reside at 2.83, 3.30, 3.63, 4.08, 4.57 and 4.80 eV, and also for 8a2, the six main peaks (x′, a′~e′) are found at 3.05, 3.45, 3.68, 4.20, 4.60 and 5.01 eV, compared with the experimental spectra of six peaks (X, A~E) of 3.06, 3.30, 3.71, 4.20, 4.61 and 5.03 eV. The conclusion can be deduced that such two types of isomers are coexisting because of the similar structures of two capped pentagonal bipyramids and close energy. For LuGe₉^-, the three peaks (x, a, b) of 3.16, 3.89 and 4.72 eV of the theoretical spectra are in excellent agreement with experimental spectra (X, A, B) of 3.23, 3.89 and 4.72 eV. For LuGe₁₀^-, the three peaks (x, a, b) positioned at 3.79, 4.31 and 4.78 eV are corresponding experimental value (X, A, B) of 4.10, 4.52 and 4.98 eV. The theoretical result has a certain red shift compared with the experimental one. For LuGe₁₁^-, the simulated spectra of three peaks marked as x, a and b are 3.60, 4.19 and 4.68 eV. In comparison with the experimental X, A and B of 3.85, 4.21 and 4.73 eV, the peaks of a and b agree well with A and B. For LuGe₁₂^-, the peaks (x, a~c) situated at 3.47, 4.35, 4.77 and 5.05 eV contrast with the experimental values (X, A~C) of 3.67, 4.51, 4.94 and 5.34 eV. The calculation result has a small deviation from the experimental one. For LuGe₁₃^-, the 13a1 and 13a2 have been presented as similar total energy isomers. The 13a1 and 13a2 have three peaks placed at 3.38, 3.80, 4.27 eV and 3.54, 3.99, 4.87 eV correlated to the experimental peaks of 3.59, 4.03 and 4.83 eV. Owing to similar half cage structures, combined with PES, 13a1 and 13a2 are the coexisting structures. For LuGe₁₄^-, three distinct peaks (x, a, b) lie in 3.63, 4.12 and 5.11 eV, which coincide with three experimental peaks (X, A, B) of 3.68, 4.13 and 5.14 eV. For LuGe₁₅^-, simulated peaks are placed at 3.93, 4.66 and 5.23 eV against experimental peaks of 3.98, 4.69 and 5.25 eV. For LuGe₁₆^-, due to stable Frank-Kasper structure, the two peaks of x and a conform with X and A at 3.96 and 4.99 eV. In much the same as LuGe₁₆^-, the LuGe₁₇^- of simulated and experimental PES exhibits four obvious peaks of 3.47 (3.47), 4.03 (4.08), 4.67 (4.70) and 5.23 (5.29) eV. For LuGe₁₈^-, its simulated PES spectra have four distinct peaks (x, a, b, c) at 3.68, 4.16, 4.93 and 5.44 eV, respectively. Except for the first peak, the last three peaks (a, b, c) reproduce good experimental peaks (A, B, C) of 4.16, 4.93 and 5.44 eV^[24]. The adjacent isomers 17a and 19a have a small bump in the low energy region of the experimental PES spectra^[24], so we infer that 18a should also have this peak. But it is not observed in the experimental PES spectra^[24]. This conclusion needs to be further verified by experiment. For LuGe₁₉^-, the three peaks (x, a, b) of 3.76, 4.15 and 5.33 eV are analogous to the experimental peaks (X, A, B) of 3.67, 4.15 and 5.33 eV.

  Figure 4

  

  Figure 4.  Simulated and experimental PES spectra of LuGe_n^- (n = 6~19) clusters

  DownLoad: Full-Size Img PowerPoint

  Theoretical calculation of adiabatic electron affinities (AEAs) and the first vertical detachment energies (VDEs) of LuGe_n clusters as well as experimental data are collected in Table 1. The calculated values of AEA and VDE are derived from AEA = E(optimized neutral) – E(optimized anion) and VDE = E(neutral at optimized anion geometry) – E(optimized anion). From Table 1, for both AEA and VDE, the experimental values of 8a2, 13a2, 14a and 17a are in good agreement with the theoretical ones with a minor difference less than 0.20 eV. The largest deviation of AEA is for LuGe₁₅, which is off by 0.55 eV. Although PES is a powerful experimental technique for measuring AEAs and, in principle, is the most accurate scheme to determine the AEAs of the corresponding neutral species, it is difficult to determine accurate AEAs if the recorded PES shows a featureless long and very rounded tail with no clear onset. The PES of LuGe₁₅^- may be one such example^[24]. In this case, theoretical calculation is necessary to help determine accurate AEAs, especially for excellent agreement between the theoretically simulated and experimental PES spectra. Therefore, we dare to predict the AEA of LuGe₁₅ is 3.73 eV rather than 3.18 eV. The theoretical VDE values of 6a, 7a, 9a, 12a, 15a, 16a and 19a are all in accord with their experimental ones with energy difference less than 0.20 eV. Besides, the calculated and experimental VDE of 10a, 11a, 12a and 18a do not match well. However, the AEA of simulation and experiment are consistent, which proves the credibility of the results from the other side. In summary, the simulated AEA and VDE outcomes of 8a2 and 13a2 are highly in line with corresponding experimental values, further proving that 8a1, 8a2 and 13a1, 13a2 are coexistence systems. By the way, LuGe₁₆^- as the most stable Frank-Kasper structure has the highest value in AEA and VDE no matter for theoretical and experimental results.

  Table 1

  

  Table 1.  Theoretical and Experimental AEA, and VDE of LuGe_n (n = 6~19)

  DownLoad: CSV

  	AEA			VDE
  Cluster	Theor.	Exp.		Theor.	Exp.
  6a	2.43	2.13 ± 0.05		2.90	2.95
  7a	2.34	2.10 ± 0.05		2.72	2.70
  8a1	2.49	2.45 ± 0.05		2.83	3.06
  8a2	2.47	2.45 ± 0.05		3.05	3.06
  9a	2.93	2.59 ± 0.05		3.16	3.23
  10a	3.09	3.29 ± 0.05		3.79	4.10
  11a	3.11	3.15 ± 0.05		3.60	3.85
  12a	3.39	2.97 ± 0.05		3.47	3.67
  13a1	3.21	3.08 ± 0.05		3.38	3.59
  13a2	3.17	3.08 ± 0.05		3.54	3.59
  14a	3.29	3.11 ± 0.05		3.63	3.68
  15a	3.73	3.18 ± 0.05		3.93	3.98
  16a	3.79	3.32 ± 0.05		3.96	3.98
  17a	3.14	3.08 ± 0.05		3.47	3.47
  18a	3.34	3.22 ± 0.05		3.68	4.16
  19a	3.52	3.25 ± 0.05		3.76	3.67

  3.3 Ionization potential

  Ionization potential (IP), including vertical ionization potential (VIP) and adiabatic ionization potential (AIP), is the important parameter in both physical and chemical properties. The VIP is described as the difference of total energies where the equation follows VIP = E(cation at optimized neutral geometry) − E(optimized neutral) and AIP defined as the difference of total energies calculated by AIP = E(optimized cation) − E(optimized neutral). The calculation results of VIP and AIP of LuGe_n (n = 6~19) are all gathered in Table 2, with no experimental data for comparison. For the sake of observation, point line chart is also presented in Fig. 5, in which the highest value of VIP for LuGe₁₅ is 7.08 eV and that of AIP for LuGe₁₆ is 6.20 eV. On the contrary, the lowest values of VIP and AIP are 5.85 eV for LuGe₆ and.61 eV for LuGe₁₁. On the whole of VIP and AIP curves, the three humps are obviously displayed in n = 7, 12 and 15 for VIP and four humps in n = 7, 9, 12 and 16 for AIP.

  $ {\text{ABE(LuG}}{{\text{e}}_n}{\text{)}} = \frac{{{\text{[}}E({\text{Lu}}) + nE({\text{Ge)}} - E({\text{LuG}}{{\text{e}}_n}){\text{]}}}}{{(n + {\text{1)}}}} $

  (0.1)

  $ {\text{ABE(LuGe}}_n^{{\text{ + 1/ - 1}}}{\text{)}} = \frac{{{\text{[}}E{\text{(Lu)}} + {\text{(}}n - {\text{1)}}E{\text{(Ge)}} + E{\text{(G}}{{\text{e}}^{{\text{ + 1/ - 1}}}}{\text{)}} - E{\text{(LuGe}}_n^{{\text{ + 1/ - 1}}}{\text{)]}}}}{{{\text{(}}n + {\text{1)}}}} $

  (0.2)

  $ {{{\Delta }}^{\text{2}}}E{\text{(LuGe}}_n^{{\text{ + 1/0/ - 1}}}{\text{)}} = E{\text{(LuGe}}_{n - 1}^{{\text{ + 1/0/ - 1}}}{\text{)}} + E{\text{(LuGe}}_{n + 1}^{{\text{ + 1/0/ - 1}}}{\text{)}} - {\text{2}}E{\text{(LuGe}}_n^{{{ + 1/0/ - 1}}}{\text{)}} $

  (0.3)

  Table 2

  

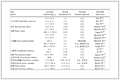  Table 2.  Vertical Ionization Potential (VIP) and Adiabatic Ionization Potential (AIP) of LuGe_n (n = 6~19)

  DownLoad: CSV

  Cluster	VIP (eV)	AIP (eV)		Cluster	VIP (eV)	AIP (eV)
  LuGe6	5.85	5.68		LuGe13	6.66	5.65
  LuGe7	6.47	6.10		LuGe14	6.25	5.79
  LuGe8	6.48	5.91		LuGe15	7.08	5.93
  LuGe9	6.49	5.94		LuGe16	6.79	6.20
  LuGe10	6.05	5.68		LuGe17	6.31	6.01
  LuGe11	6.48	5.61		LuGe18	6.34	6.14
  LuGe12	6.81	5.76		LuGe19	6.57	6.13

  Figure 5

  

  Figure 5.  Ionization potential of LuGe_n (n = 6~19) clusters

  DownLoad: Full-Size Img PowerPoint

  3.4 Stabilities

  To investigate the relative stabilities of the lowest energy structure of LuGe_n^(+/0/-) (n = 6~19), the average bonding energy (ABE) and second energy difference (Δ²E) are calculated by equations (1.1)~(1.3) in which E is the total energies related to atom or compound, and HOMO-LUMO gap (E_gap) is derived from the energy of the lowest unoccupied molecular orbital minus the highest occupied molecular orbital. All are exhibited in Fig. 6.

  Figure 6

  

  Figure 6.  Graphical representation of (a) Size dependence of the average bond energy (ABE), (b) Second energy difference (Δ²E) and (c) HOMO-LUMO energy gap (E_gap) of the ground state LuGe_n^(+/0/-) (n = 6~19)

  DownLoad: Full-Size Img PowerPoint

  From Fig. 6a, it is clear to see that the ABE values of the neutral cluster are all lower than the others, which indicates the cation and anion are more stable because both of them have closed-shell electronic configuration which has increased their stabilities. The ABE value of LuGe₁₆^- is 3.54 which is higher than the other anionic and neutral clusters. The reason can be described that not only LuGe₁₆^- is Frank-Kasper endohedral motif with high T_d symmetry, but also has the closed-shell electronic configuration as super atom with ultra-stability. Owing to LuGe₁₆^-, the neutral and cationic LuGe₁₆ are also more stable than the other ones of the same type because both those structures are derived from Frank-Kasper structure with different degrees of distortion.

  The second-order difference in energy of LuGe_n^(+/0/-) (n = 6~19) is shown in Fig. 6b, which is to evaluate the relative stability of such cluster and its two directly adjacent ones. For anion, the three peaks of anionic type are located at LuGe₉^-, LuGe₁₂^- and LuGe₁₆^-. For the neutral, the obvious four peaks are situated at LuGe₉, LuGe₁₁, LuGe₁₃ and LuGe₁₆. For cation, LuGe₈⁺, LuGe₁₁⁺ LuGe₁₃⁺ and LuGe₁₇⁺ have four peaks. That means such clusters are more stable than the adjacent ones.

  The E_gap is an important physical parameter for semiconductors to evaluate not only the chemical reactivity but also the optical properties. As can be seen in Fig. 6c, the apparent two peaks of LuGe₁₀^- and LuGe₁₆^- are about 2.41 and 2.55 eV. As far as we know, such energy gap value is larger enough for the luminescent host from ground states to excited states^[45]. Besides the proper energy gap for luminescent materials, that is also suitable for catalysis material. As photo catalyst, it is very important for visible light response, so that the materials with adjustable energy gaps can be achieved^[46]. As the LuGe₁₉⁺ has the lowest energy gap of 1.06 eV to LuGe₁₆^- of 2.55 eV, it can fit within the visible light range. In summary, these clusters as an excellent building block are potentially applied to multi-functional nanomaterials.

  3.5 Iso-chemical shielding surface of LuGe₁₆^-

  To further understand the stability of LuGe₁₆^- Frank-Kasper structure, the iso-chemical shielding surface (ICSS) based on the real-space function which is similarly related to the nucleus-independent chemical shift (NICS) is calculated by gauge-independent atomic orbital (GIAO) method, and the results are analyzed by Multiwfn code^{[47, 48]}. Compared to NICS, the ICSS can exhibit the entire three-dimensional space of chemical shielding against the external magnetic field as well as different isosurfaces drawn at different iso-values to clearly reveal the shielding or deshielding effect from the delocalized electron. From Fig. 7a, the red region is the shielding area with isovalue of 0.05 ppm and the blue region is deshielding area with isovalue of 0.05 ppm. Due to the high symmetry of the LuGe₁₆^- cluster, the shielding areas are exhibited by three protruding red areas, which indicates that the inner region of the cluster has strong magnetic shielding. Owing to the electron lone pair existence, it presents high electron delocalization and aromaticity. Fig. 7b displays the ICSS value from the distance of 0.4 to 3.0 Å, which reveals that the shielding value of the inner cage is larger than that of the outer surface. The maximum shielding value about 83.97 ppm is located at a distance of 1 Å from the center. In conclusion, the measurement of ICSS can be used for the aromatic properties of the LuGe₁₆^- cluster, which shows the key factors of its stability.

  Figure 7

  

  Figure 7.  ICSS of LuGe₁₆^- cluster. (a) Isosurface of ICSS with isovalue of 0.05 ppm (red region) and –0.05 ppm (blue region), (b) ICSS curve map of magnetic shielding value with distance from center

  DownLoad: Full-Size Img PowerPoint

  3.6 Chemical bonding analysis and density of states of the LuGe₁₆^- cluster

  In order to gain insight and understand the better thermosdynamic and chemical stability of the LuGe₁₆^- cluster with Frank-Kasper structure, the adaptive natural density partitioning (AdNDP) method, based on the natural bond orbital (NBO) developed by Zubarev and Boldyrev, is used to study the cluster as a multicenter bonding system^[49]. For example, nc-2e is denoted as n from the range 1 to the number of atoms in the cluster system. According to Fig. 8, the LuGe₁₆^- cluster of chemical bonding of 68 valence electrons with T_d symmetry can be split into three parts: lone pair, 2c-2e, and 4c-2e. The Ge atom, which is located on each of the four triad axes, has a lone pair. Besides the four Ge atoms on the triad axes, the remaining Ge₁₂ cage is attributed to 18 2c-2e localized Ge–Ge σ bonds possessing 1.87~1.89 electrons in each bond. The last ones are categorized into 12 delocalized 4c-2e σ bonds connecting the cage core atom of Lu to the outer Frank-Kasper Ge₁₆ skeleton, which can stabilize the entire structure and reveals the full encapsulated LuGe₁₆^- endohedral cluster.

  Figure 8

  

  Figure 8.  AdNDP analysis of LuGe₁₆^- cluster. ON is the occupation number

  DownLoad: Full-Size Img PowerPoint

  For more clarity regarding the unique electronic structure of LuGe₁₆^- cluster, the calculated density of states and partial density of states are analyzed and shown in Fig. 9. It can be clearly seen that 6s, 5d, 5p, and 5s orbitals of Lu combined with 4s and 4p orbitals of Ge contribute to the main density of states of LuGe₁₆^- cluster. The 4f orbitals are isolated at –3~–4 eV region. Near the Fermi level (dot line), the main contribution is from 5d orbital of Lu and 4p orbital of Ge. In other words, the d-orbital of Lu is mainly participating in hybridization with Ge orbital to stabilize the cluster. Moreover, the bottom part of the conduction band mainly consists of 5d orbitals of Lu, and 4s, 4p and 4d orbitals of Ge. In the context of the AdNDP and DOS analyses, the orbital sequence of LuGe₁₆^- can be represented as 1S²1P⁶1D¹⁰1F¹⁴2S²1G¹⁸2P⁶2D¹⁰, a jellium model for 68 valence electrons.

  Figure 9

  

  Figure 9.  Calculated and labelled total density of states (TDOS) and partial density of states (PDOS) of most stable LuGe₁₆^- cluster

  DownLoad: Full-Size Img PowerPoint

  3.7 UV-Vis spectra of LuGe₁₆^- cluster

  The UV-Vis spectra are an essential parameter for luminescent and photocatalytic materials. The most stable LuGe₁₆^- cluster is selected as a model structure due to its significant stability and appropriate energy gap. Time-dependent DFT (TDDFT) calculations are performed at the level of TPSSh/aug-cc-pVTZ for Ge and ECP28WMB for Lu in order to analyze transitions in 120 excited states which are enough to describe. The curves are arranged by Gaussian broadening function with the full width at half maximum (FWHM) about 0.20 eV. Fig. 10 shows that the four peaks are positioned at 334, 369, 408 and 540 nm. The peak of 334 nm has a maximum absorption intensity and its 95% contribution can be decomposed into three parts of S₀ → S₁₁₅ with contribution of 8%, S₀ → S₁₀₇ with contribution of 72% and S₀ → S₁₀₄ with contribution of 15%. The peak of 369 nm with 92% comes from the S₀ → S₇₄. The peak of 408 nm mainly has 12% contribution of S₀→S₅₈ and 69% of S₀ → S₃₅. The last peak of 540 nm is comprised of 46% contribution of S₀ → S₆ and 53% contribution of S₀ → S₁₂. The absorption band is in the range of 300~600 nm and most of it falls in the visible light region, which can be excited by natural light. Meanwhile, the strong absorption bands are situated in the blue and near-ultraviolet regions. In other words, the LuGen₁₆^- cluster with high stability can be further explored as possible optoelectronic material.

  Figure 10

  

  Figure 10.  Calculated UV-Vis spectra of LuGe₁₆^- cluster. Solid and dotted lines represent the absorption curve and oscillator strength, respectively

  DownLoad: Full-Size Img PowerPoint

  4. CONCLUSION

  The growth behavior, thermodynamic stabilities, chemical activities, and electronic properties of cationic, neutral, and anionic lutetium doped germanium cluster LuGe_n^(+/0/-) (n = 6~19) are calculated by the ABCluster unbiased global search technique with a TPSSh-hybrid density functional theory. In terms of experimental PES spectra, AEA and VDE compared with the simulations, the growth pattern of anionic LuGe_n^- can be depicted from adsorbed (n = 6~9) to the link structure (n = 10 ~ 12), then to half cage (n = 13~14), finally to the cage-like motif (n = 15~19). For the neutral and cationic LuGe_n clusters, the growth characteristics are less evident than anions. The neutral LuGe_n forms the link structure when n = 12~14 and when n = 15, those can be formed as cage-like structures. For cations, the link structure begins at n = 11 and lasts until n = 15, then creates cage-like motif. The stability analysis shows the great performance for LuGe₁₆^-, and the reason can be described not only by its unique Frank-Kasper framework with high T_d symmetry without any imaginary frequency, but also by the specific electronic configurations. To further support that discovery, the aromaticity, density of states and AdNDP analysis are set. The ICSS results show the LuGe₁₆^- possesses the aromatic nature with a max value of 83.97 ppm. Furthermore, the 3D isosurface of ICSS also proves the strong magnetic shielding effect in the cage inner. The AdNDP results exhibit that the orbital sequence 1S²1P⁶1D¹⁰1F¹⁴2S²1G¹⁸2P⁶2D¹⁰ matches 68 electrons forming as a closed-shell superatomic model. Existence of lone pair increases the delocalization of LuGe₁₆^-, which is also beneficial for the cluster stability. Finally, the density of states presents the 5d orbitals of Lu hybridized with 4p orbital of Ge to further stabilize the cluster of LuGe₁₆^-. Owing to the excellent stability and proper energy gap of LuGe₁₆^- and others, the lutetium doped germanium clusters are possible candidates to fabricate a number of assembled optoelectronic materials.

  
  
• 1. [1]

     Pillarisetty, R. Academic and industry research progress in germanium nanodevices. Nature 2011, 479, 324−328. doi: 10.1038/nature10678

  2. [2]

     Vaughn II, D. D.; Schaak, R. E. Synthesis, properties and applications of colloidal germanium and germanium-based nanomaterials. Chem. Soc. Rev. 2013, 42, 2861−2879. doi: 10.1039/C2CS35364D

  3. [3]

     Cardoso, J.; Marom, S.; Mayer, J.; Modi, R.; Podestà, A.; Xie, X.; van Huis, M. A.; Di Vece, M. Germanium quantum dot grätzel-type solar cell. Phys. Status. Solidi. A 2018, 215, 1800570−1800577. doi: 10.1002/pssa.201800570

  4. [4]

     Jia, L.; Fan, G.; Zi, W.; Ren, X.; Liu, X.; Liu, B.; Liu, S. Ge quantum dot enhanced hydrogenated amorphous silicon germanium solar cells on flexible stainless steel substrate. Sol. Energy 2017, 144, 635−642. doi: 10.1016/j.solener.2017.01.042

  5. [5]

     Adachi, S.; Takahashi, T. Photoluminescent properties of K₂GeF₆: Mn⁴⁺ red phosphor synthesized from aqueous HF/KMnO₄ solution. J. Appl. Phys. 2009, 106, 13516−13521. doi: 10.1063/1.3160303

  6. [6]

     Zhou, Q.; Zhou, Y.; Liu, Y.; Luo, L.; Wang, Z.; Peng, J.; Yan, J.; Wu, M. A new red phosphor BaGeF₆: Mn⁴⁺: hydrothermal synthesis, photo-luminescence properties, and its application in warm white LED devices. J. Mater. Chem. C 2015, 3, 3055−3059. doi: 10.1039/C4TC02956A

  7. [7]

     Hu, W.; Cong, H.; Huang, W.; Huang, Y.; Chen, L.; Pan, A.; Xue, C. Germanium/perovskite heterostructure for high-performance and broadband photodetector from visible to infrared telecommunication band. Light-Sci. Appl. 2019, 8, 1−10. doi: 10.1038/s41377-018-0109-7

  8. [8]

     Liu, Y.; Li, C.; Li, B.; Song, H.; Cheng, Z.; Chen, M.; He, P.; Zhou, H. Germanium thin film protected lithium aluminum germanium phosphate for solid-state Li batteries. Adv. Energy Mater. 2018, 8, 1702374−1702380. doi: 10.1002/aenm.201702374

  9. [9]

     Zhao, J.; Du, Q.; Zhou, S.; Kumar, V. Endohedrally doped cage clusters. Chem. Rev. 2020, 120, 9021−9163. doi: 10.1021/acs.chemrev.9b00651

  10. [10]

      Jena, P.; Sun, Q. Super atomic clusters: design rules and potential for building blocks of materials. Chem. Rev. 2018, 118, 5755−5870. doi: 10.1021/acs.chemrev.7b00524

  11. [11]

      Kumar, V.; Kawazoe, Y. Metal-encapsulated caged clusters of germanium with large gaps and different growth behavior than silicon. Phys. Rev. Lett. 2002, 88, 235504−235507. doi: 10.1103/PhysRevLett.88.235504

  12. [12]

      Kumar, V.; Kawazoe, Y. Metal-encapsulated fullerenelike and cubic caged clusters of silicon. Phys. Rev. Lett. 2001, 87, 45503−45506. doi: 10.1103/PhysRevLett.87.045503

  13. [13]

      Zhou, S.; Zhao, Y.; Zhao, J. Cage clusters: from structure prediction to rational design of functional nanomaterials. Chin. J. Struct. Chem. 2020, 39, 1185−1193.

  14. [14]

      Lasmi, M.; Mahtout, S.; Rabilloud, F. The effect of palladium and platinum doping on the structure, stability and optical properties of germanium clusters: DFT study of PdGe_n and PtGe_n (n = 1~20) clusters. Comput. Theor. Chem. 2020, 1181, 112830−112836. doi: 10.1016/j.comptc.2020.112830

  15. [15]

      Borshch, N. A.; Kurganskii, S. I. Spatial structure and electron energy spectrum of HfGe_n^- (n = 6~20) clusters. Inorg. Mater. 2015, 51, 870−876. doi: 10.1134/S0020168515080075

  16. [16]

      Bandyopadhyay, D.; Sen, P. Density functional investigation of structure and stability of Ge_n and Ge_nNi (n = 1~20) clusters: validity of the electron counting rule. J. Phys. Chem. A 2010, 114, 1835−1842. doi: 10.1021/jp905561n

  17. [17]

      Jaiswal, S.; Kumar, V. Growth behavior and electronic structure of neutral and anion ZrGe_n (n = 1~21) clusters. Comput. Theor. Chem. 2016, 1075, 87−97. doi: 10.1016/j.comptc.2015.11.013

  18. [18]

      Hou, X.; Gopakumar, G.; Lievens, P.; Nguyen, M. T. Chromium-doped germanium clusters CrGe_n (n = 1~5): geometry, electronic structure, and topology of chemical bonding. J. Phys. Chem. A 2007, 111, 13544−13553.

  19. [19]

      Triedi, R. K.; Bandyopadhyay, D. Insights of the role of shell closing model and NICS in the stability of NbGe_n (n = 7~18) clusters: a first-principles investigation. J. Mater. Sci. 2019, 54, 515−528. doi: 10.1007/s10853-018-2858-3

  20. [20]

      Pham, L. N.; Nguyen, M. T. Insights into geometric and electronic structures of VGe₃^–/0 clusters from anion photoelectron spectrum assignment. J. Phys. Chem. A 2017, 121, 6949−6956. doi: 10.1021/acs.jpca.7b07459

  21. [21]

      Tang, C.; Liu, M.; Zhu, W.; Deng, K. Probing the geometric, optical, and magnetic properties of 3d transition-metal endohedral Ge₁₂M (M = Sc~Ni) clusters. Comput. Theor. Chem. 2011, 969, 56−60. doi: 10.1016/j.comptc.2011.05.012

  22. [22]

      Borshch, N. A.; Pereslavtseva, N. S.; Kurganskii, S. I. Spatial structure and electron energy spectra of ScGe_n^- (n = 6~16) clusters. Russ. J. Phys. Chem. B 2015, 9, 9−18. doi: 10.1134/S1990793115010030

  23. [23]

      Qin, W.; Lu, W.; Xia, L.; Zhao, L.; Zang, Q.; Wang, C. Z.; Ho, K. M. Structures and stability of metal-doped Ge_nM (n = 9, 10) clusters. Aip. Adv. 2015, 5, 67159−67167. doi: 10.1063/1.4923316

  24. [24]

      Atobe, J.; Koyasu, K.; Furuse, S.; Nakajima, A. Anion photoelectron spectroscopy of germanium and tin clusters containing a transition- or lanthanide-metal atom; MGe_n^- (n = 8~20) and MSn_n^- (n = 15~17) (M = Sc~V, Y~Nb, and Lu~Ta). Phys. Chem. Chem. Phys. 2012, 14, 9403−9410. doi: 10.1039/c2cp23247b

  25. [25]

      Frank, F. C.; Kasper, J. S. Complex alloy structures regarded as sphere packings. I. Definitions and basic principles. Acta. Crystallogr. 1958, 11, 184−190. doi: 10.1107/S0365110X58000487

  26. [26]

      Zhang, J.; Dolg, M. ABCluster: the artificial bee colony algorithm for cluster global optimization. Phys. Chem. Chem. Phys. 2015, 17, 24173−24181. doi: 10.1039/C5CP04060D

  27. [27]

      Zhang, J.; Dolg, M. Global optimization of clusters of rigid molecules using the artificial bee colony algorithm. Phys. Chem. Chem. Phys. 2016, 18, 3003−3010. doi: 10.1039/C5CP06313B

  28. [28]

      Frisch, M. J.; Trucks, G. W.; Schlegel, H. B.; Scuseria, G. E.; Robb, M. A.; Cheeseman, J. R.; Scalmani, G.; Barone, V.; Mennucci, B.; Petersson, G. A.; Nakatsuji, H.; Caricato, M.; Li, X.; Hratchian, H. P.; Izmaylov, A. F.; Bloino, J.; Zheng, G.; Sonnenberg, J. L.; Hada, M.; Ehara, M.; Toyota, K.; Fukuda, R.; Hasegawa, J.; Ishida, M.; Nakajima, T.; Honda, Y.; Kitao, O.; Nakai, H.; Vreven, T.; Montgomery, J. A. Jr.; Peralta, J. E.; Ogliaro, F.; Bearpark, M.; Heyd, J. J.; Brothers, E.; Kudin, K. N.; Staroverov, V. N.; Kobayashi, R.; Normand, J.; Raghavachari, K.; Rendell, A.; Burant, J. C.; Iyengar, S. S.; Tomasi, J.; Cossi, M.; Rega, N.; Millam, J. M.; Klene, M.; Knox, J. E.; Cross, J. B.; Bakken, V.; Adamo, C.; Jaramillo, J.; Gomperts, R.; Stratmann, R. E.; Yazyev, O.; Austin, A. J.; Cammi, R.; Pomelli, C.; Ochterski, J. W.; Martin, R. L.; Morokuma, K.; Zakrzewski, V. G.; Voth, G. A.; Salvador, P.; Dannenberg, J. J.; Dapprich, S.; Daniels, A. D.; Farkas, Ö.; Foresman, J. B.; Ortiz, J. V.; Cioslowski, J.; Fox, D. J. Gaussian 09, revision C. 01; Gaussian, Inc. : Wallingford, CT 2009.

  29. [29]

      Dolg, M.; Stoll, H.; Savin, A.; Preuss, H. Energy-adjusted pseudopotentials for the rare earth elements. Theor. Chim. Acta 1989, 75, 173−194. doi: 10.1007/BF00528565

  30. [30]

      Cao, X.; Dolg, M. Valence basis sets for relativistic energy-consistent small-core lanthanide pseudopotentials. J. Chem. Phys. 2001, 115, 7348−7355. doi: 10.1063/1.1406535

  31. [31]

      Cao, X.; Dolg, M. Segmented contraction scheme for small-core lanthanide pseudopotential basis sets. J. Mol. Struc-Theochem. 2002, 581, 139−147. doi: 10.1016/S0166-1280(01)00751-5

  32. [32]

      Tozer, D. J.; Handy, N. C. Improving virtual Kohn-Sham orbitals and eigenvalues: application to excitation energies and static polarizabilities. J. Chem. Phys. 1998, 109, 10180−10189. doi: 10.1063/1.477711

  33. [33]

      Akola, J.; Manninen, M.; Hakkinen, H.; Landman, U.; Li, X.; Wang, L. Photoelectron spectra of aluminum cluster anions: temperature effects and ab initio simulations. Phys. Rev. B 1999, 60, R11297−R11300. doi: 10.1103/PhysRevB.60.R11297

  34. [34]

      Kresse, G.; Hafner, J. Ab initio molecular dynamics for liquid metals. Phys. Rev. B 1993, 47, 558−561. doi: 10.1103/PhysRevB.47.558

  35. [35]

      Kresse, G.; Hafner, J. Ab initio molecular-dynamics simulation of the liquid-metal-amorphous-semiconductor transition in germanium. Phys. Rev. B 1994, 49, 14251−14269. doi: 10.1103/PhysRevB.49.14251

  36. [36]

      Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169−11186. doi: 10.1103/PhysRevB.54.11169

  37. [37]

      Kresse, G.; Furthmüller, J. Efficiency of ab-initio total energy calculations for metals and semiconductors using a plane-wave basis set. Comp. Mater. Sci. 1996, 6, 15−50. doi: 10.1016/0927-0256(96)00008-0

  38. [38]

      Perdew, J. P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865−3868. doi: 10.1103/PhysRevLett.77.3865

  39. [39]

      Blochl, P. E. Projector augmented-wave method. Phys. Rev. B 1994, 50, 17953−17979. doi: 10.1103/PhysRevB.50.17953

  40. [40]

      Kresse, G.; Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 1999, 59, 1758−1775.

  41. [41]

      Lu, T.; Chen, F. Multiwfn: a multifunctional wavefunction analyzer. J. Comput. Chem. 2012, 33, 580−592. doi: 10.1002/jcc.22885

  42. [42]

      Humphrey, W.; Dalke, A.; Schulten, K. VMD: visual molecular dynamics. J. Mol. Graph. 1996, 14, 33−38. doi: 10.1016/0263-7855(96)00018-5

  43. [43]

      Wang, J.; Wang, G.; Zhao, J. Structure and electronic properties of Ge_n (n = 2~25) clusters from density-functional theory. Phys. Rev. B 2001, 64, 205411−205415. doi: 10.1103/PhysRevB.64.205411

  44. [44]

      Chen, M.; Jiao, Y.; Luo, H.; Liu, J.; Zhang, Q. A density functional study for the isomers of germanium clusters Ge₁₁. Chin. J. Struct. Chem. 2004, 23, 227−231.

  45. [45]

      Wang, B.; Lin, H.; Huang, F.; Xu, J.; Chen, H.; Lin, Z.; Wang, Y. Non-rare-earth BaMgAl_10-2xO₁₇: xMn⁴⁺, xMg²⁺: a narrow-band red phosphor for use as a high-power warm w-LED. Chem. Mater. 2016, 28, 3515−3524. doi: 10.1021/acs.chemmater.6b01303

  46. [46]

      Li, G.; Wang, B.; Wang, R. g-C₃N₄/Ag/GO composite photocatalyst with efficient photocatalytic performance: synthesis, characterization, kinetic studies, toxicity assessment and degradation mechanism. Chin. J. Struct. Chem. 2020, 39, 1675−1688.

  47. [47]

      Klod, S.; Kleinpeter, E. Ab initio calculation of the anisotropy effect of multiple bonds and the ring current effect of arenes-application in conformational and configurational analysis. J. Chem. Soc. Perk. T. 2 2001, 1893−1898.

  48. [48]

      Schleyer, P. V. R.; Maerker, C.; Dransfeld, A.; Jiao, H.; van Eikema Hommes, N. J. R. Nucleus-independent chemical shifts: a simple and efficient aromaticity probe. J. Am. Chem. Soc. 1996, 118, 6317−6318. doi: 10.1021/ja960582d

  49. [49]

      Zubarev, D. Y.; Boldyrev, A. I. Developing paradigms of chemical bonding: adaptive natural density partitioning. Phys. Chem. Chem. Phys. 2008, 10, 5207−5217. doi: 10.1039/b804083d

• 

  Figure 1  The lowest-energy structures of LuGe_n^- (n = 6~19) with point group. The blue and red balls represent the Ge and Lu atoms, respectively

  下载: 全尺寸图片 幻灯片
  

  Figure 2  The lowest-energy structures of LuGe_n (n = 6~19) with point group. The blue and red balls represent the Ge and Lu atoms, respectively

  下载: 全尺寸图片 幻灯片
  

  Figure 3  The lowest-energy structures of LuGe_n⁺ (n = 6~19) with point group. The blue and red balls represent the Ge and Lu atoms, respectively

  下载: 全尺寸图片 幻灯片
  

  Figure 4  Simulated and experimental PES spectra of LuGe_n^- (n = 6~19) clusters

  下载: 全尺寸图片 幻灯片
  

  Figure 5  Ionization potential of LuGe_n (n = 6~19) clusters

  下载: 全尺寸图片 幻灯片
  

  Figure 6  Graphical representation of (a) Size dependence of the average bond energy (ABE), (b) Second energy difference (Δ²E) and (c) HOMO-LUMO energy gap (E_gap) of the ground state LuGe_n^(+/0/-) (n = 6~19)

  下载: 全尺寸图片 幻灯片
  

  Figure 7  ICSS of LuGe₁₆^- cluster. (a) Isosurface of ICSS with isovalue of 0.05 ppm (red region) and –0.05 ppm (blue region), (b) ICSS curve map of magnetic shielding value with distance from center

  下载: 全尺寸图片 幻灯片
  

  Figure 8  AdNDP analysis of LuGe₁₆^- cluster. ON is the occupation number

  下载: 全尺寸图片 幻灯片
  

  Figure 9  Calculated and labelled total density of states (TDOS) and partial density of states (PDOS) of most stable LuGe₁₆^- cluster

  下载: 全尺寸图片 幻灯片
  

  Figure 10  Calculated UV-Vis spectra of LuGe₁₆^- cluster. Solid and dotted lines represent the absorption curve and oscillator strength, respectively

  下载: 全尺寸图片 幻灯片

  Table 1.  Theoretical and Experimental AEA, and VDE of LuGe_n (n = 6~19)

  	AEA			VDE
  Cluster	Theor.	Exp.		Theor.	Exp.
  6a	2.43	2.13 ± 0.05		2.90	2.95
  7a	2.34	2.10 ± 0.05		2.72	2.70
  8a1	2.49	2.45 ± 0.05		2.83	3.06
  8a2	2.47	2.45 ± 0.05		3.05	3.06
  9a	2.93	2.59 ± 0.05		3.16	3.23
  10a	3.09	3.29 ± 0.05		3.79	4.10
  11a	3.11	3.15 ± 0.05		3.60	3.85
  12a	3.39	2.97 ± 0.05		3.47	3.67
  13a1	3.21	3.08 ± 0.05		3.38	3.59
  13a2	3.17	3.08 ± 0.05		3.54	3.59
  14a	3.29	3.11 ± 0.05		3.63	3.68
  15a	3.73	3.18 ± 0.05		3.93	3.98
  16a	3.79	3.32 ± 0.05		3.96	3.98
  17a	3.14	3.08 ± 0.05		3.47	3.47
  18a	3.34	3.22 ± 0.05		3.68	4.16
  19a	3.52	3.25 ± 0.05		3.76	3.67

  下载: 导出CSV

  Table 2.  Vertical Ionization Potential (VIP) and Adiabatic Ionization Potential (AIP) of LuGe_n (n = 6~19)

  Cluster	VIP (eV)	AIP (eV)		Cluster	VIP (eV)	AIP (eV)
  LuGe6	5.85	5.68		LuGe13	6.66	5.65
  LuGe7	6.47	6.10		LuGe14	6.25	5.79
  LuGe8	6.48	5.91		LuGe15	7.08	5.93
  LuGe9	6.49	5.94		LuGe16	6.79	6.20
  LuGe10	6.05	5.68		LuGe17	6.31	6.01
  LuGe11	6.48	5.61		LuGe18	6.34	6.14
  LuGe12	6.81	5.76		LuGe19	6.57	6.13

  下载: 导出CSV
•