English

• 1. INTRODUCTION

  Decreasing the emission of CO₂ is currently a global concerned serious problem. Scientists continually explore a new energy to solve the problem. Hydrogen is a clean-burning fuel to replace fossil fuel^[1]. Hydrogen mainly exists in the form of H₂. Transporting H₂ through pipelines is the most effective way relative to other transportation methods^{[2, 3]}. Hydrogen embrittlement of pipeline steels is an extremely important topic which reduces the mechanical properties of pipeline steel^[4]. The gas pipelines always require welding to assemble each other for long distance transmission. Hydrogen embrittlement on welded joint and welding heat affected zone becomes complex because of the welding residual stress and microstructure heterogeneity. Welding residual stress on the surface has been shown to change surface reactivity in a significant way^{[5, 6]}. Adsorption of hydrogen is sensitive to surface stress. The ductility decrease of X80, X100 steel during tensile test in hydrogen environment mainly occurs in necking stage^[7]. Some scientists attributed this phenomenon to surface stress state turning into biaxial stress^[8]. Abundant dislocations slip along Fe(110) during plastic deformation. New Fe(110) surface appears on the surface.

  Hydrogen adsorption is the first step to penetrate into steel. For the past few years, adsorption of hydrogen on Fe(110) surface has been extensively investigated by means of experimental and theoretical methods. Some information has been confirmed, such as adsorption energy, adsorption site and adsorption configuration^[9-13]. Jiang first studied H atom adsorption on Fe(110) by spinpolarized density functional theory calculation^[14]. He confirmed that the adsorption structure and adsorption energy agree with experiment. Urslaan found that quasi-threefold site is the most stable adsorption position with adsorption energy of 3.0 eV per hydrogen atom^[15]. The surface layer space increases after the adsorption of hydrogen, which facilitates hydrogen atoms diffusing into the Fe bulk.

  Adsorbed H atom penetrating into Fe bulk involves three steps. First, H atom diffuses between the adsorption sites on the surface. Second, H atom enters the gap between surface atoms and the second layer atoms. Third, H atom diffuses between gaps in bulk^[16]. H atom diffuses on Fe surface with a barrier of 1.9 kcal/mol. The energy barrier from surface to subsurface is 9.5 kcal/mol^{[14, 17]}. The diffusion energy barriers from the second to the third layer decreases, which is almost identical to the barrier in bulk Fe. The step that hydrogen atom penetrates into the subsurface is the most difficult. It is the key step in determining the velocity of hydrogen atom penetrating into Fe bulk^{[18, 19]}. Some scientists studied hydrogen diffusion in strained Fe bulk^{[20, 21]}. But the effect of surface strain on hydrogen diffusion into subsurface has not been reported.

  The above-mentioned work focused on the unstrained Fe surface. It is a new way to control adsorption of gas on many metal surfaces by changing surface strain^[22-24]. Tensile strain increases the binding strength of CO on the Pt surface^[25]. Oxygen atom adsorbs preferentially in the regions of expanded Cu(111) surface^[26]. However, there is no theoretical study on hydrogen atom adsorption and diffusion on the strained Fe surface. The theoretical calculations on hydrogen adsorption and diffusion at strained Fe(110) surface are of great significance to investigate the relationship between hydrogen embrittlement and surface strain. H adsorption and diffusion on the strained Fe(110) surface were studied by employing density function theory to explore the mechanism biaxial strain hydrogen embrittlement in this paper.

  2. COMPUTATION DETAILS AND MODELS

  Our first principles calculation was carried out by the Cambridge Serial Total Energy Package (CASTEP) based on density functional theory (DFT). The Kohn-Sham equation with a plane wave basis set was solved by employing three-dimensional periodic boundary conditions^[27]. Generalized gradient approximation (GGA) of revised Perdew Burke Ernzerhof (rPBE) functional was applied for the treatment electron exchange-correlation term. All calculations were done in the spin polarized. We tested kinetic energy cutoff and k-point sampling for the calculated principle cells. The kinetic energy cut off energy of 425 eV was used. A uniform k-point sampling of a 19 × 19 × 19 k-mesh for Fe principle cell was employed^[28]. The equilibrium lattice constant a = 2.845 Å was obtained. It agrees well with the experiment value (2.866 Å)^[29] and previous calculations of 2.834 Å^[30].

  The Fe(110) surface has been studied in this work, since it is the new surface during plastic deformation. The Fe(110) surface model was represented by a seven layers slab model and a 2× 2 unit cell which has been used in other articles^{[14, 31]}. A 12 Å depth vacuum was placed on the model to ensure separation. Uniform k point was used on the Monkhorst-Pack and grid size of 7 × 7 × 1. BFGS algorithm was used to relax the slab model. The upper three layers of Fe atoms were allowed to relax, and the other bottom layers were fixed to represent bulk under the surface.

  There are four different coordinated sites on Fe(110). They are short bridge (Sb), on-top (Top), long bridge (Lb) and pseudo three-fold hollow (Tf), as present in Fig. 1. Hydrogen atom was then adsorbed on the four coordinated sites. The H atom and the upper three layers of Fe atoms were allowed to relax while the other bottom Fe atoms were constrained. The adsorption energy E_ads for the four sites was calculated by Equation 1.

  $ E_{\text {ads }}=E_{\mathrm{H}+\mathrm{slab}}-\left(E_{\text {slab }}+E_{\mathrm{H}}\right) $

  (1)

  Figure 1

  

  Figure 1.  Coordinated sites of Fe(110). On top of the Fe atom (top), short bridge (Sb), long bridge (Lb), pseudo-threefold-coordinated hollow (Tf). Red and blue balls represent surface and subsurface atoms, respectively. Fe1, Fe2, Fe3 and Fe4 in the surface and Fe5, Fe6, Fe7 and Fe8 in second layer are neighboring Fe atoms

  DownLoad: Full-Size Img PowerPoint

  Where E_H is the energy of a single H atom, E_slab and E_H+slab are the total energy of the slab without and with an adsorbed hydrogen atom. E_H is calculated by putting a H atom in a cubic box with 12 Å side and carrying out 1 × 1 × 1 k point calculation. The negative value of E_ads means that the adsorption of H atom releases heat. The more negative E_ads illustrates more binding strength between the slab and H. The surface strain varies from –5% to 5%. Lattice constants of 2.702 Å, 2.788 Å, 2.845 Å and 2.902 Å, 2.987 Å correspond to 5% compressive strain, 2% compressive strain, no strain, 2% tensile strain, and 5% tensile strain, respectively.

  Complete LST/QST calculation was used to find the minimum energy path for H atom diffusion from preferentially adsorbed site to the gap between surface and subsurface. Linear synchronous transit (LST) maximization was performed, followed by repeated conjugate gradient minimizations and quadratic synchronous transit (QST) maximizations until a transition state was found. The force acting on atoms was minimized to 0.03 eV/Å for the geometry optimization in the LST/QST calculation.

  3. RESULTS AND DISCUSSION

  3.1 Hydrogen adsorption on the Fe(110) surface

  The H-surface distance, the H atom adsorption energy, and the distance from H atom to its nearest Fe atom neighbor are shown in Table 1. It is shown that the adsorption energy of hydrogen is negative. This illustrates the process of H adsorption is exothermic whether the Fe(110) surface is strained or not. The adsorption energy for the unstrained surface coincides well with the previous calculations^[32]. Tf site is found to be the most negative for all strained and unstrained surfaces. The adsorption energy of the Lb site is similar to that of the Tf site, which agrees well with the experiment^[33]. Hydrogen atom prefers to be adsorbed at the high-coordinated site. Other researchers confirmed that the Tf site is a true minimum, whereas all the other sites exhibit imaginary frequency^[15]. The distance of 0.95 Å between H and the surface at Tf agrees well with previous calculations (0.91 Å)^[34]. The hydrogen atom on Tf site binds with the largest number of Fe atoms. The hydrogen atom on Tf site maintains the maximum distance to the nearest Fe atoms, but keeps the shortest distance to the Fe(110) surface. Lb site is virtually a low coordinated site because Lb site at the Fe(110) surface matches to top site of the second layer Fe atom. Therefore, the adsorption energy on Lb site is not the most negative.

  Table 1

  

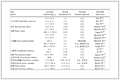  Table 1.  Adsorption Energy of Hydrogen (E_ads), Adsorption Height (h), and Distance from Hydrogen to the Nearest Neighboring Fe (d_Fe–H)

  DownLoad: CSV

  Lattice strain	Lb				Sb				Tf				Top
  	Eads	h	dFe–H		Eads	h	dFe–H		Eads	h	dFe-H		Eads	h = dFe–H
  –5%	–2.72	0.96	1.75		–2.61	1.18	1.71		–2.91	0.97	1.77		~	~
  –2%	–2.73	0.95	1.75		–2.62	1.17	1.70		–2.95	0.96	1.77		~	~
  0	–2.74	0.95	1.74		–2.63	1.16	1.69		–2.98	0.95	1.79		–1.98	1.57
  2%	–2.75	0.94	1.74		–2.64	1.14	1.65		–3.02	0.93	1.78		~	~
  5%	–2.77	0.94	1.73		–2.69	1.14	1.65		–3.12	0.92	1.78		~	~
  Distances are in units of Å, and adsorption energies are in units of eV.

  H atom on the top site of the strained surface moves to the tf site after configuration is relaxed. H atom can not be adsorbed on top site when surface is strained. Chohan^[15] found that top site was a rank-two saddle point for two imaginary frequencies. H atom on top site lives the farthest away from Fe(110) surface, which indicates the instability of H adsorption on the Top site.

  The adsorbed hydrogen atoms relax towards the surface on Tf, Sb and Lb sites when lattice is expanded. It demonstrates tensile strain reduces the distance between adsorbed H atom and surface. In the studied strain range, the binding strength of H atom at Tf site is stronger than other sites with the most negative E_ads. The adsorption energy becomes more negative with increasing the tensile strain. This demonstrates that lattice expansion enhances the binding strength between the H atom and Fe(110) surface. However, compressive strain makes the adsorption energy less negative. This means the binding strength between H atom and the surface becomes weaker. Lattice compression is expected to be a way to suppress hydrogen adsorption on the Fe surface.

  3.2 Hydrogen diffusion into the Fe(110) subsurface

  Complete LST/QST calculation is a reliable method to find the diffusion path. It was performed to find transition state and the minimum energy pathways of hydrogen diffusion from surface to subsurface. As calculated above, H prefers to be adsorbed at the Tf site on Fe(110) which was defined as the initial state. Hydrogen atom prefers to be in the tetrahedral interstice site of Fe bulk^[30]. Finial state can be considered as hydrogen atom occupying tetrahedral interstice site between the surface and subsurface Fe atoms, as shown in the inset of Fig. 2.

  Figure 2

  

  Figure 2.  Minimum energy pathways for hydrogen diffusion from surface to subsurface on the unstrained surface

  DownLoad: Full-Size Img PowerPoint

  The diffusion activation energy E_a is defined as the energy deference between the transition state and initial state. It is calculated by Eq. 2 where E_TS is the energy of transition state and E_IS is that of the initial state.

  $ E_{\mathrm{a}}=E_{\mathrm{TS}}-E_{\mathrm{IS.}} $

  (2)

  Diffusion paths of H atom on the unstrained and strained Fe(110) surfaces were calculated. Fig. 2 shows the diffusion path of H atom on the unstrained Fe(110) surface. Transition state configuration of unstrained system is presented in the inset of Fig. 2. Transition state is found that H atom is in a distorted tetrahedron which is almost identical as the final state. Diffusion path does not change with respect to strain variation, and it is similar to that of unstrained Fe(110). Transition state configuration is not sensitive to surface strain. Fig. 3 shows the diffusion activation energy as the function of strain. Surface strain varies the diffusion barrier. H atom has to overcome 0.85, 1.05 and 1.27 eV into subsurface for 5% tensile strained, 0 strained, and –5% strained surface respectively. The diffusion energy barrier for 0 strain in this calculation coincides with Jiang's calculation 1.02 eV^[30].

  Figure 3

  

  Figure 3.  Strain dependence of diffusion activation energy

  DownLoad: Full-Size Img PowerPoint

  Tensile strain decreases diffusion energy barrier and accelerates H diffusion into Fe bulk. On the contrary, compressive strain suppresses H diffusion into subsurface. Tetrahedron gap volume in the transition state was calculated based on the coordinates of four Fe atoms, as shown in Fig. 4. There is more tetrahedron gap volume in the transition state of expanded surface. It is responsible for the lower diffusion energy barrier. Smaller tetrahedron gap volume of compressive Fe(110) surface increases the diffusion energy barrier.

  Figure 4

  

  Figure 4.  Strain dependence of the tetrahedral gap volume

  DownLoad: Full-Size Img PowerPoint

  3.3 Electronic structure of H/Fe(110) system

  Partial density of states (PDOS) of H atom and the nearest neighbor Fe atom were calculated to investigate the orbital interaction for H adsorbed on the Tf site. Fig. 5 shows projected density of states of H and Fe atoms on the unstrained surface before and after hydrogen adsorption. Adsorption of H atom obviously changes the PDOS profiles. The orbital of H 1s hybridizes with Fe 4s, 3p and 3d states^[28]. There is a sharp peak to clean H atom around 0 eV. H 1s shifts down to –6.1 eV and becomes broader after adsorption due to the strong H 1s and Fe 4s hybridization and a little hybridization with Fe 3p and 3d.

  Figure 5

  

  Figure 5.  Projected density of states before and after H adsorption on the surface. (a) is for the clean H atom, (b) for the clean Fe atom on the first layer, and (c) for the nearest Fe with H atom

  DownLoad: Full-Size Img PowerPoint

  Fig. 6 shows PDOS evolutions of Fe 4s, Fe 3p, Fe 3d and H 1s orbitals with respect to strain variation. The orbital hybridization between Fe 4s and H 1s is still dominant on different strained surface, whereas H 1s orbital hybridizes a little with Fe 3p and Fe 3d orbitals. The PDOS of H 1s and Fe 4s shift right to higher energy, which results in strong binding between H and Fe atoms with strain increase. The similar feature of PDOS dependence on strain has been observed on the Mg[0001] surface^[35].

  Figure 6

  

  Figure 6.  Strain dependence of projected density of states of (a) Fe 4s, (b) Fe 3p and (c) Fe 3d of the nearest neighboring Fe atom, and (d) H 1s on the Tf site

  DownLoad: Full-Size Img PowerPoint

  Mulliken populations of the adsorbed H atom on Tf site and the nearest neighboring Fe atom with respect to the strain variation were calculated to further analyze the orbital hybridization, with the results shown in Table 2. Surface strain alters the orbital occupation of clean surface and H adsorbed system. When the surface is compressed, the Mulliken electrons of Fe 4s and Fe 3d orbitals decrease, and Mulliken electrons of Fe 3p increase. When surface strain changes from 5% to –5%, the Mulliken electrons of Fe 4s orbital are decreased by 0.1 e on the clean surface and 0.08 e on the H adsorbed surface. The loss of Fe 4s is due to the gain of Fe 3p. It demonstrates that electrons transfer from Fe 4s to Fe 3p when surface strain varies from elongation to compression. Previous PDOS analysis shows the binding interaction between Fe and H results from the hybridization of H 1s and Fe 4s. Electrons transfer from s to d on compressive surface, which weakens the binding strength between H and Fe atoms. The electron donation from Fe to H atom is observed. The population analysis shows that the charges transfer to H from the Fe atom on 5%, 0 and –5 strained surfaces are 0.31, 030, and 0.29 e, respectively. More electrons are donated when the surface is expanded.

  Table 2

  

  Table 2.  Mulliken Population for H 1s, Fe 4s, Fe 3p and Fe 3d Orbitals for the Tf and Clean Surface as the Function of Strain

  DownLoad: CSV

  	Lattice strain	Mulliken population
  		H 1s	Fe 4s	Fe 3p	Fe 3d
  	5%		0.81	0.56	6.65
  Clean surface	0		0.75	0.66	6.63
  	–5%		0.71	0.75	6.59
  	5%	1.31	0.76	0.51	6.66
  Tf	0	1.30	0.70	0.63	6.63
  	–5%	1.29	0.64	0.73	6.60

  The charge transfer between Fe surface and H on tf site was investigated by charge density difference. The charge density difference is defined as Δρ_Fe+H(ε) = ρ_Fe+H(ε) – ρ_Fe(ε) – ρ_H where ρ_Fe+H(ε) is the total charge density of H adsorbed on Fe surface, and ρ_H and ρ_Fe(ε) are the charge density of isolated H atom and clean Fe surface, respectively. Red region in Fig. 7 represents negative charge center, and blue region shows the positive charge center. H atom gains extra electrons (in red), wheras Fe surface loses electrons (in blue). The region of H atom when ε = 5% is more red than others. It demonstrates more eletrons are transferred from Fe to the H atom, which concides well with the above results of Mulliken population analysis.

  Figure 7

  

  Figure 7.  Charge density difference of H adsorption on the Tf site when ε = 5% (a), ε = 0 (b), ε = –5% (c)

  DownLoad: Full-Size Img PowerPoint

  4. CONCLUSION

  Employing the first principles techniques, atomic H adsorption and diffusion on biaxial strain strained Fe(110) surface were studied. We have found that the Tf site is the most stable on compressive and tensile surfaces. H atom can not adsorb on the top site of the strained surface. Our calculation reveals that H atom becomes less adsorptive when compressive strain is applied to the Fe(110) surface. The analysis of the projected density of states shows that H 1s hybridizes with Fe 4s orbital. Electrons are transferred from the Fe to H atom. Compressive strain reduces the transferred electrons and decreases the Mulliken electrons of Fe 4s orbital, which weakens the binding interaction between H and Fe atoms. H atom diffuses from Tf site into subsurface through a distorted tetrahedron. Surface strain does not change diffusion path but affect the diffusion barrier energy. Tetrahedron gap volume in the transition state of –5% compressive strain system reduces, which increases the diffusion barrier. This suggests compressive strain impedes H penetrating into the Fe subsurface.

  The present results indicate that the surface strain is a factor to control adsorption and diffusion of hydrogen on Fe(110) surface. There are residual and working stresses in welded joint and welding heat affected the zone of pipeline steel. Tensile strain decreases the distance between adsorbed hydrogen atom and surface, and increases the binding strength, and accelerates hydrogen atom penetrating into Fe(110). However, compressive strain has the opposite effect on adsorption and diffusion of hydrogen atom. To decrease hydrogen embrittlement of pipeline, it is necessary to take measures to decrease surface tensile stress and apply compressive stress on the surface. This is meaningful to further study the effect of surface stress on hydrogen embrittlement.

  
  
• 1. [1]

     Seyitoglu, S. S.; Dincer, I.; Kilicarslan, A. Energy and energy analyses of hydrogen production by coal gasification. Int. J. Hydrogen Energy 2017, 42, 2592−2600. doi: 10.1016/j.ijhydene.2016.08.228

  2. [2]

     Nanninga, N. E.; Slifka, A.; Levy, Y. A review of fatigue crack growth for pipeline steels exposed to hydrogen. J. Res. Nat. Inst. Stand. Technol. 2010, 115, 437−439. doi: 10.6028/jres.115.030

  3. [3]

     Dodds, P. E.; Demoullin, S. Conversion of the UK gas system to transport hydrogen. Int. J. Hydrogen. Energy 2013, 38, 7189−7200. doi: 10.1016/j.ijhydene.2013.03.070

  4. [4]

     Briottet, L.; Batisse, R.; Dinechin, G. D. Recommendations on X80 steel for the design of hydrogen gas transmission pipelines. Int. J. Hydrogen Energy 2012, 37, 9423−9430. doi: 10.1016/j.ijhydene.2012.02.009

  5. [5]

     Gsell, M.; Jakob, P.; Menzel, D. Effect of substrate strain on adsorption. Science 1998, 5364, 717−720. doi: 10.3321/j.issn:1003-8728.1998.05.010

  6. [6]

     Jakob, P.; Gsell, M.; Menzel, D. Interactions of adsorbates with locally strained substrate lattices. Chin. J. Chem. Phys. 2001, 114, 10075−10085. doi: 10.1063/1.1369161

  7. [7]

     Xie, W.; Peng, L.; Peng, D. Processes of H₂ adsorption on Fe(110) surface a density functional theory study. Appl. Surf. Sci. 2014, 296, 47−52. doi: 10.1016/j.apsusc.2014.01.028

  8. [8]

     Nanninga, N. E.; Levy, Y. S.; Drexler, E. S. Comparison of hydrogen embrittlement in three pipeline steels in high pressure gaseous hydrogen environments. Corros. Sci. 2012, 59, 1−9. doi: 10.1016/j.corsci.2012.01.028

  9. [9]

     Huo, C. F.; Li, Y. W.; Wang, J. G. Surface structure and energetics of hydrogen adsorption on the Fe(111) surface. J. Phys. Chem. B 2005, 109, 14160−14167. doi: 10.1021/jp051907s

  10. [10]

      Amaya, R. S.; Linares, D. H.; Duarte, H. A. Effect of hydrogen in adsorption and direct dissociation of CO on Fe(100) surface a DFT study. J. Anal. Chem. 2015, 6, 38-46. doi: 10.4236/ajac.2015.61004

  11. [11]

      Juan, A.; Hoffmann, R. Hydrogen on the Fe(110) surface and near bulk bcc Fe vacancies: a comparative bonding study. Surf. Sci. 1999, 421, 1−16. doi: 10.1016/S0039-6028(98)00780-8

  12. [12]

      Kunisada, Y.; Sakaguchi, N. Effects of hydrogen atoms on vacancy formation at fcc Fe(111) Surfaces. J. Jap. Ins. Met. A 2015, 79, 447−451. doi: 10.2320/jinstmet.JAW201508

  13. [13]

      Wang, T.; Tian, X.; Yang, Y. Co-adsorption and mutual interaction of nCO + mH₂ on the Fe(110) and Fe(111) surfaces. Catal. Today 2015, 261, 82−92.

  14. [14]

      Jiang, D. E.; Carter, E. A. Adsorption and diffusion energetics of hydrogen atoms on Fe(110) from first principles. Surf. Sci. 2003, 547, 85−98. doi: 10.1016/j.susc.2003.10.007

  15. [15]

      Chohan, U. K.; Jimenez, M. E.; Koehler, S. P. K. Surface atomic relaxation and magnetism on hydrogen-adsorbed Fe(110) surfaces from first principles. Appl. Surf. Sci. 2016, 387, 385−392. doi: 10.1016/j.apsusc.2016.06.027

  16. [16]

      Sanchez, J.; Fullea, F.; Andrade, M. C. Ab-initio molecular dynamics simulation of hydrogen diffusion in α-iron. Phys. Rev. B 2010, 81, 132102−132111. doi: 10.1103/PhysRevB.81.132102

  17. [17]

      Sorescu, D. C. First principles calculations of the adsorption and diffusion of hydrogen on Fe(100) surface and in the bulk. Catal. Today 2005, 105, 44−65. doi: 10.1016/j.cattod.2005.04.010

  18. [18]

      Chohan, U. K.; Koehler, S. P. K.; Jimenez, M. E. Diffusion of hydrogen into and through γ-iron by density functional theory. Surf. Sci. 2018, 672, 56−61.

  19. [19]

      Wen, X.; Bai, P.; Han, Z. Effect of vacancy on adsorption/dissociation and diffusion of H₂S on Fe(110) surfaces a density functional theory study. Appl. Surf. Sci. 2019, 465, 833−845. doi: 10.1016/j.apsusc.2018.09.220

  20. [20]

      Li, X. Hydrogen diffusion in aFe under an applied 3-axis strain a quantum manifestation. Int. J. Hydrogen Energy 2015, 40, 10340−10345. doi: 10.1016/j.ijhydene.2015.06.089

  21. [21]

      Li, S. H.; Wang, Y.; Zhao, W. M. Influence of single axis strain on site occupation and diffusion of hydrogen atom in αFe. Acta. Phys. Sin. 2017, 66, 193−198.

  22. [22]

      Goikoetxea, I.; Juaristi, J.; Muiño, R. D. Surface strain improves molecular adsorption but hampers dissociation for N₂ on the Fe/W(110) surface. Phys. Rev. Lett. 2014, 113, 066103−066108. doi: 10.1103/PhysRevLett.113.066103

  23. [23]

      Jeon, J.; Yu, B. D.; Hyun, S. Surface strain effects on the adsorption and the diffusion of Au atoms on MgO(001) surfaces. J. Kor. Phy. Soc. 2016, 69, 1776−1780. doi: 10.3938/jkps.69.1776

  24. [24]

      Yang, L.; Shu, D. J.; Li, S. C. Influence of strain on water adsorption and dissociation on rutile TiO₂(110) surface. Phys. Chem. 2016, 18, 14833−14839.

  25. [25]

      Kuwabara, A.; Saito, Y.; Koyama, Y. First principles calculation of CO and H₂ adsorption on strained Pt surface. Mater. Trans. Jim. 2008, 49, 608−616.

  26. [26]

      Xu, Y.; Mavrikakis, M. Adsorption and dissociation of O₂ on Cu(111) thermochemistry reaction barrier and the effect of strain. Surf. Sci. 2001, 494, 131−144. doi: 10.1016/S0039-6028(01)01464-9

  27. [27]

      Clark, S. J.; Segall, M. D.; Pickard, C. J. First principles methods using CASTEP. Zei. Kri. 2005, 220, 567−570.

  28. [28]

      Zhang, F. C.; Li, C. F.; Wen, P.; Luo, Q.; Ran, Z. L. First principles investigation of interaction between interstitial hydrogen atom and Fe metal. Acta Phys. Sin. 2014, 63, 227101−227111 doi: 10.7498/aps.63.227101

  29. [29]

      Nichtl, P. W.; Gossmann, J.; Hammer, L. Adsorption of hydrogen on Fe(110) at cryogenic temperatures investigated by low energy electron diffraction. J. Vac. Sci. Tec. Vac. Sur. Fil. 1992, 10, 501−507. doi: 10.1116/1.578179

  30. [30]

      Jiang, D. E.; Carter, E. A. Diffusion of interstitial hydrogen into and through bcc Fe from first principles. Phys. Rev. B 2004, 70, 064102−064106. doi: 10.1103/PhysRevB.70.064102

  31. [31]

      Jiang, D. E.; Carter, E. A. Carbon atom adsorption on and diffusion into Fe(110) and Fe(100) from first principles. Phys. Rev. B 2005, 71, 045402−045409.

  32. [32]

      Cremaschi, P.; Yang, H.; Whitten, J. L. Ab initio chemisorption studies of H on Fe(110). Sur. Sci. 1995, 330, 255−264. doi: 10.1016/0039-6028(95)00244-8

  33. [33]

      Moritz, W.; Imbihl, R.; Behm, R. J. Adsorption geometry of hydrogen on Fe(110). J. Chem. Phys. 1985, 83, 1959−1968. doi: 10.1063/1.449334

  34. [34]

      Shen, X.; Chen, J.; Sun, Y. M. Hydrogen diffusion on Fe surface and into subsurface from first principles. Surf. Sci. 2016, 654, 48−55. doi: 10.1016/j.susc.2016.08.005

  35. [35]

      Lei, H.; Wang, C.; Yao, Y. Strain effect on the adsorption, diffusion, and molecular dissociation of hydrogen on Mg(0001) surface. J. Chem. Phys. 2013, 139, 224702−224709. doi: 10.1063/1.4839595

• 

  Figure 1  Coordinated sites of Fe(110). On top of the Fe atom (top), short bridge (Sb), long bridge (Lb), pseudo-threefold-coordinated hollow (Tf). Red and blue balls represent surface and subsurface atoms, respectively. Fe1, Fe2, Fe3 and Fe4 in the surface and Fe5, Fe6, Fe7 and Fe8 in second layer are neighboring Fe atoms

  下载: 全尺寸图片 幻灯片
  

  Figure 2  Minimum energy pathways for hydrogen diffusion from surface to subsurface on the unstrained surface

  下载: 全尺寸图片 幻灯片
  

  Figure 3  Strain dependence of diffusion activation energy

  下载: 全尺寸图片 幻灯片
  

  Figure 4  Strain dependence of the tetrahedral gap volume

  下载: 全尺寸图片 幻灯片
  

  Figure 5  Projected density of states before and after H adsorption on the surface. (a) is for the clean H atom, (b) for the clean Fe atom on the first layer, and (c) for the nearest Fe with H atom

  下载: 全尺寸图片 幻灯片
  

  Figure 6  Strain dependence of projected density of states of (a) Fe 4s, (b) Fe 3p and (c) Fe 3d of the nearest neighboring Fe atom, and (d) H 1s on the Tf site

  下载: 全尺寸图片 幻灯片
  

  Figure 7  Charge density difference of H adsorption on the Tf site when ε = 5% (a), ε = 0 (b), ε = –5% (c)

  下载: 全尺寸图片 幻灯片

  Table 1.  Adsorption Energy of Hydrogen (E_ads), Adsorption Height (h), and Distance from Hydrogen to the Nearest Neighboring Fe (d_Fe–H)

  Lattice strain	Lb				Sb				Tf				Top
  	Eads	h	dFe–H		Eads	h	dFe–H		Eads	h	dFe-H		Eads	h = dFe–H
  –5%	–2.72	0.96	1.75		–2.61	1.18	1.71		–2.91	0.97	1.77		~	~
  –2%	–2.73	0.95	1.75		–2.62	1.17	1.70		–2.95	0.96	1.77		~	~
  0	–2.74	0.95	1.74		–2.63	1.16	1.69		–2.98	0.95	1.79		–1.98	1.57
  2%	–2.75	0.94	1.74		–2.64	1.14	1.65		–3.02	0.93	1.78		~	~
  5%	–2.77	0.94	1.73		–2.69	1.14	1.65		–3.12	0.92	1.78		~	~
  Distances are in units of Å, and adsorption energies are in units of eV.

  下载: 导出CSV

  Table 2.  Mulliken Population for H 1s, Fe 4s, Fe 3p and Fe 3d Orbitals for the Tf and Clean Surface as the Function of Strain

  	Lattice strain	Mulliken population
  		H 1s	Fe 4s	Fe 3p	Fe 3d
  	5%		0.81	0.56	6.65
  Clean surface	0		0.75	0.66	6.63
  	–5%		0.71	0.75	6.59
  	5%	1.31	0.76	0.51	6.66
  Tf	0	1.30	0.70	0.63	6.63
  	–5%	1.29	0.64	0.73	6.60

  下载: 导出CSV
•