English

• 1. INTRODUCTION

  The value of harnessing the power of energetic materials (EMs) has been realized for quite some time, resulting in their pervasive use in different commercial processes^[1-3]. Advancements in energetic materials have also been driven by a need to find more powerful, stable, and reliable materials for military devices. These traditional energetic compounds are explored such as trinitrotoluene (TNT), pentaerythritol tetranitrate (PETN), cyclotrimethylenetrinitramine (RDX), cyclotetramethylene tetranitramine (HMX), 1, 1-diamino-2, 2-dinitroethylene (FOX-7) and triaminotrinitrobenzene (TATB). They consist of organic C–H–N–O molecules which combine both fuel (C–H backbone) and oxidizer (nitro (NO₂) or nitrate (NO₃)) groups within a single mole cule^[4]. Afterwards, much work has been focused on the derivatives of these traditional high energy density materials (HEDMs). Although such investigations provide some important results, the demands of high energy and insensitivity are quite often contradictory to each other, making the development of novel HEDMs a challenging problem. Thus, systemic molecular design for high-nitrogen compound is still needed to explore novel insensitive HEDMs.

  As we all know that these compounds containing triazole ring, as an important class of high-energy density materials (HEDMs), have received vital attention both in military and civilian applications^{[5, 6]}. Recently, Yang research group conduct the study on synthesis of different neutral compounds consisting of 1, 2, 3-2H-triazole and 1, 2, 4-triazole rings carrying energetic moieties like amino, nitroimino, nitro as well as azo^[7]. Unfortunately, their outstanding properties such as high density, high positive heat of formation (HOF) and excellent detonation properties seem to be contrary to the stability and sensitivity. Thus, in order to overcome this difficulty, one possible approach is to replace one hydrogen atom using different high-energy groups to design different derivatives. Thus, we choose excellent 4-nitro-5-(5-nitro-1, 2, 4-triazol-3-yl)-2H-1, 2, 3-triazolate among these synthetic organic molecules as the initial material to develop energetic materials. So, in our work, twelve kinds of energetic groups (NO₂, NH₂, NHNH₂, NHNO₂, NNH₂NO₂, NNO₂NO_2, N₃, ONO₂, NNO₂ONO₂, OH, NF₂ and C(NO₂)₃) are introduced to this framework using computer simulation, and generate a series of high energy materials. Computational studies can provide understanding relationships between molecular structure and property, and make it possible to screen candidate compounds.

  2. CALCULATION METHODS

  Numerous researches have shown that the DFT-B3LYP method in combination with 6-311G** basis set can give accurate energies, molecular structures and physicochemical properties especially for the high energy density materials (HEDMs)^[8-11]. Thus, the Gaussian 09 package^[12] of theoretical chemistry was used in this paper under B3LYP/6-311G** level of theory to conduct our work. In our study, we used isodesmic reactions for calculating the HOF of the title molecules at 298 K as follows^[8]:

  $ {{\text{C}}_{\text{4}}}{{\text{H}}_{\text{2}}}{{\text{O}}_{\text{4}}}{{\text{N}}_{\text{9}}} - {\text{R}} + {\text{C}}{{\text{H}}_{\text{4}}} = {{\text{C}}_{\text{4}}}{{\text{H}}_{\text{3}}}{{\text{O}}_{\text{4}}}{{\text{N}}_{\text{9}}} + {\text{C}}{{\text{H}}_{\text{4}}} - {\text{R}} $

  (1)

  Where R is NO₂, NH₂, NHNH₂, NHNO₂, NNH₂NO₂, NNO₂NO_2, N₃, ONO₂, NNO₂ONO₂, OH, NF₂, C(NO₂)₃ (See Fig. 1). For isodesmic reaction, gas-phase HOF at 298 K can be written as the following formula:

  $ {\Delta}{H_{298}} = {\Delta}{E_0} + {\Delta}ZPE + {\Delta}HT + {\Delta}nRT $

  (2)

  Figure 1

  

  Figure 1.  All molecular structures of the title derivatives

  DownLoad: Full-Size Img PowerPoint

  Gas-phase HOF(g) usually misestimates the detonation properties of energetic compounds, so for accurately predicting the detonation performance of new high organic molecules. It is also necessary to calculate the HOF in solid phase, which can be obtained from its value in the gas phase by using the Hess' law^[13]:

  $ {\Delta}{H_f}{\text{(}}s{\text{)}} = {\Delta}{H_f}{\text{(}}g{\text{)}} - {\Delta}Hsub $

  (3)

  where $ {\Delta}Hsub $ is the heat of sublimation. This one can be calculated using the below equation proposed by Rice and Politzer et al^{[14, 15]}:

  $ {\Delta}H_{sub}^{} = {\alpha _1}{{\text{(}}SA{\text{)}}^2} + {\beta _1}{{\text{(}}\upsilon {\sigma ^2}_{tot}{\text{)}}^{0.5}} + {\lambda _1} $

  (4)

  where SA is the molecular surface area; σ_tot² is an indicator of the variability of the electrostatic potential on the molecular surface; ν is the degree of balance between the positive and negative potentials on the molecular surface; $ {\alpha _1} $, $ {\beta _1} $ and $ {\gamma _1} $ are the fitting coefficients from Refs. 14 and 15.

  The important factors of an explosive, including the detonation velocity and pressure, were evaluated by the extensively used empirical Kamlet-Jacob (K-J)^[16] expression according to the calculated density and the enthalpy of formation:

  $ D = 1.01{{\text{(}}N{\overline M ^{1/2}}{Q^{1/2}}{\text{)}}^{1/2}}{\text{(}}1 + 1.30\rho 0{\text{)}} $

  (5)

  $ P = 1.558{\left( {{\rho _0}} \right)^2}N{\bar M^{1/2}}{Q^{1/2}} $

  (6)

  where, P is the detonation pressure (GPa), D is the detonation velocity (km/s), and$ \rho 0 $is the packed density (g/cm³). N is the moles of detonation gases produced per gram explosive and M is the average molecular weight of these gases. Besides, Q is the heat of detonation (cal/g), N, $ \bar M $and Q can be obtained based on Table 1. It is well known that the density is very critical to the explosive properties, and accurate calculation of crystal density is of much difficulty in Politzer's previous study^[17]. The equation for predicting the density of neutral systems is:

  $ {\text{Crystal density}} \rho = \alpha {\text{(}}M/V{\text{m)}} + \beta {\text{(}}\nu {\sigma ^2}tot{\text{)}} + \gamma $

  (7)

  Table 1

  

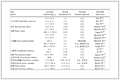  Table 1.  Calculated Methods for the Values of N, $ \bar M $, and Q of Explosive C_aH_bO_cN_d. $ \bar M $ is the Molecular Weight in g/mol and$ \Delta H{f^0} $the Solid Phase HOF in kcal/mol

  DownLoad: CSV

  Parameters	Explosives components conditions
  	c≥2a + b/2	2a + b/2 > c≥b/2	b/2 > c
  $ N $	(b + 2c + 2d)/4M	(b + 2c + 2d)/4M	(b + d)/2M
  $ \bar M $	4M/(b + 2c + 2d)	(56d + 88c-8b)/(b + 2c + 2d)	(2b + 28d + 32c)/(b + d)
  Q×10-3	(28.9b + 94.05a + 0.239$ {\Delta}H{f^0} $)/M	[28.9b + 94.05(c/2-b/4) + 0.239$ {\Delta}H{f^0} $]/M	(57.8c + 0.239$ {\Delta}H{f^0} $)/M

  when we checked for accuracy about the equation, it can be found the$ \rho $value may be closer to the experimental data based on the actual situation in this work. Therefore, we replaced$ \rho 0 $with$ \rho $to precisely predict the density of molecules, M is the molecular mass and V_m is the van der Waals volume; $ \beta $ and$ \gamma $are correction constants from ref^[17].

  What's more, the impact sensitivity is another crucial parameter. The impact sensitivity test involves subjecting a sample to the impact of the standard mass falling from different heights. Despite the boundedness and uncertainties associated with the impact test, there are many methods to predict impact sensitivity through different molecular properties. Nowadays, we recommend a wide equation proposed by Pospíšil et al.^[18]:

  Model 1: $ {H_{50}} = {\alpha _2}\sigma _ + ^2 + {\beta _2}\nu + {\lambda _2} $

  where the values of coefficients$ {\alpha _2} $, $ {\beta _2} $, and$ {\gamma _2} $ are taken from Ref. 18. In order to consider comprehensively other factors to the impact sensitivity, we also used other models of H₅₀ equation^[19]:

  Model 2: $ H50 = a1 + a2\exp \left[ - \right.(a3\left| {\bar V{s^ + } - \left| {\bar Vs} \right.} \right.\left. {^ - } \right\|\left. ) \right] $

  where $ \bar V{s^ + } $and $ \bar V{s^{\text{ - }}} $ are the respective averages of the positive and negative regions of the electrostatic potential (ESP), where the best fit parameters are$ a1 $= 9.1949 cm, $ a2 $= 803.4464 cm, and$ a3 $= 0.3663 (kcal/mol)^-1.

  Model 3: $ {H_{50}} = a1 + a2\exp (a3v) $

  where $ a1 $= 29.3248 cm, $ a2 $= 0.001386 cm, and $ a3 $= 48.8381.

  Model 4: $ {H_{50}} = a1 + a2\exp \left[ { - a3(Q - a4)} \right] $

  The corresponding parameters are $ a1 $= 27.8331 cm, $ a2 $= 0.1135 cm, $ a3 $= 11.0793 (kcal/g)^-1, $ a4 $= 1.6606 kcal/g, and Q means the heat of detonation.

  Model 5: $ {H_{50}} = a1\exp \left[ {a2v - a3(Q - a4)} \right] $

  where parameters corresponding to the best fit of this function to the data are $ a1 $= 1.3410 cm, $ a2 $= 8.1389, $ a3 $= 6.7922 (kcal/g)^-1, and $ a4 $= 1.4737 kcal/g.

  3. RESULTS AND DISCUSSION

  3.1 Heats of formation

  The basic structures are presented in Fig. 1. For the sake of discussion, all derivatives of 4-nitro-5-(5-nitro-1, 2, 4-triazol-3-yl)-2H-1, 2, 3-triazolate are named A~L in sequence. Heat of formation (HOF) is a significant factor to reflect the energy content of a compound and molecular stability. High and positive HOF means high energy but less stability. The value of HOF (kJ/mol) is calculated based on formulas (2), (3) and (4), and the ultimate results are listed in Table 2. At the same time, Table 2 also lists the total energies (E₀, a.u), zero-point energies (ZPE, a.u) and thermal corrections (H_T, a.u) in the isodesmic reactions at the B3LYP/6-311G** level of theory. Besides, Table 2 presents the data of traditional energetic materials to compare our results in this section.

  Table 2

  

  Table 2.  Calculated Total Energies (E₀, a.u.), Zero-point Energies (ZPE, a. u.), Thermal Corrections (H_T, a. u.), and Solid Phase Heats of Formation (HOFs, Solid, kJ/mol) of Twelve New High Explosives Compared to Traditional Explosives

  DownLoad: CSV

  Compound	E0 (a.u.)	ZPE (a.u.)	HT (a.u.)	HOFs (kJ/mol)
  Parent				559.59
  A	−1096.99510	0.10545	0.01582	560.54
  B	−947.83270	0.12144	0.01438	636.49
  C	−1003.16217	0.13797	0.01572	727.30
  D	−1152.35009	0.12294	0.01681	585.48
  E	−1207.69207	0.13888	0.01832	639.04
  F	−1356.85629	0.12292	0.01980	551.73
  G	−1055.91629	0.10359	0.01623	1341.05
  H	−1172.18564	0.10926	0.01682	470.28
  I	−1432.04593	0.12635	0.02093	470.54
  J	−967.67723	0.10831	0.01396	504.66
  K	−1146.21206	0.10244	0.01606	533.47
  L	−1545.38885	0.13719	0.02230	326.75
  TNTa				−63.12
  RDXa				79.00
  HMXa				75.24
  FOX-7b				−133.70
  CL-20a				377.04
  a Data from Ref. [21]. b Data from Ref. [15].

  As shown in Table 2, all derivatives of the title compound have positive HOFs, which is one of the requirements for energetic materials^[20]. It is worth noting that not only all derivatives exhibit excellent HOFs but also all the corresponding values (326.75~1341.05 kJ/mol) are higher compared with TNT (–63.12 kJ/mol), RDX (79.00 kJ/mol), HMX (75.24 kJ/mol) and FOX-7 (–133.70 kJ/mol)^{[15, 21]}. These values, especially for compound G, meet most military and civilian requirements. The HOFs decrease to the lowest value (326.75 kJ/mol) when about three nitroso groups are present on the substitution site. The HOFs then obtain maximum (1341.05 kJ/mol) as additional -N₃ group is added to the original skeleton because abundant N–N bonds also have positive effect for increasing the HOFs value. When the substituent is NO₂, NH₂, NHNH₂, NHNO₂, NNH₂NO₂ or N₃, an increase HOF value of its substituted compounds is large when compared with the unsubstituted case. While the substituent is NNO₂NO₂, ONO₂, NNO₂ONO₂, OH, NF₂ or C(NO₂)₃, the case is quite different. As expected, the introduction of nitrogen rich groups (NO₂, NH₂, NHNH₂, NHNO₂, NNH₂NO₂, N₃) results in higher heats of formation than their parent (559.59 kJ/mol), which means these designed materials are promising to apply in the future. This change trend of HOF can be arranged in the sequence G > C > E > B > D > A > F > K > J > I > H > L.

  3.2 Molecular structures and electronic properties

  Our method to design new high energy density materials on years of experience is coupled with interdisciplinary computational way in this field. There are three concrete standards that a newly-designed energetic explosive should meet: (i) stable five-membered heterocyclic rings obtained in our designed structures in order to stop abrupt dissociation/decomposition of these rings, (ii) a relatively-high number of nitrogen and oxygen atoms to guarantee lower oxygen balance (OB) and higher heat of formation (HOF), (iii) a combination of amino and nitro groups to improve stability to mechanical impact and thermal stability of the final materials. What's more, one advantage of these compounds designed by us is that each new organic molecule contains two heterocycles, which greatly improves the stability of the materials.

  The highest occupied molecular orbitals (HOMOs) and the lowest unoccupied molecular orbitals (LUMOs) are called frontier molecular orbitals (FMOs). The energy gap ($ \Delta ELUMO - HOMO $) between HOMO and LUMO reflects the chemical reactivity, kinetic stability and optical polarizability of the compounds. The molecular frontier orbital energy levels and their gaps are contained in Table 3. It is obvious to see from Table 3 that HOMO energies vary from −0.3027 to −0.3438 a.u. and LUMO from −0.1106 to −0.1602 a.u. for all new designed derivatives, and the frontier orbital energies differ from different compounds. Except for compound J, it is noteworthy that all the investigated molecules have higher energy gap than their parent and compound K with a NF₂ group has the maximum value. Thus, the contributions of the NF₂ group to the derivatives improve the stability of the compound and reduce their chemical activity. As shown in Table 3, the value of the investigated molecule J is less than the parent, indicating that the new designed molecule may be more sensitive than the parent. The differences predict that the chemical activity of the title compounds decreases in the following order: K > C > F > D > A > I > E > L > H > B > G > J. The low value of$ \Delta ELUMO - HOMO $ means this electron transfer is easy from HOMO to LUMO. In other words, these molecules are more sensitive and chemically active and less stable.

  Table 3

  

  Table 3.  Frontier Orbital Energies and Their Differences of Title Compounds at the B3LYP/6-311G** Level of Theory

  DownLoad: CSV

  Compound	HOMO	LUMO	$ \Delta ELUMO - HOMO $
  Parent	−0.3036	−0.13928	0.1644
  A	−0.3369	−0.1441	0.1929
  B	−0.3027	−0.1266	0.1761
  C	−0.3192	−0.1160	0.2032
  D	−0.3228	−0.1278	0.1950
  E	−0.3094	−0.1226	0.1868
  F	−0.3360	−0.1385	0.1975
  G	−0.3257	−0.1602	0.1655
  H	−0.3128	−0.13441	0.1784
  I	−0.3223	−0.1313	0.1910
  J	−0.3053	−0.1465	0.1589
  K	−0.3371	−0.1325	0.2046
  L	−0.3438	−0.1577	0.1860

  3.3 Impact sensitivity

  Apart from the energies and structures, the sensitivity of energetic materials is also a key study of keen interest to researchers in the research area of high energy density materials. Here, we use characteristic height (H₅₀) to evaluate the sensitivity of molecules. Theoretical prediction of impact sensitivity for energetic materials has long been considered a difficult study, because the sensitivity of organic compounds is relevant to their decomposition kinetics and thermodynamics, which is very complicated. Actually, a calculation using crystal volume factors to assess impact sensitivities of nitramine energetic is proposed by Politzer and co-workers, which give acceptable accuracy^[18]. So, the impact sensitivities of new organic derivatives were also computed by using model 1 for H₅₀ values. Besides, according to previous study, some researchers found that the compounds are stabilized by the delocalization of electronic charge. The impact sensitivity can be relevant to the degree of charge separation and the presence of strongly positive electrostatic potential maxima on the molecular surface^[19]. Thus, in order to further discuss whether our system fits the relevant models, we also calculate the H₅₀ values of the other four models in this work. The H₅₀ values of all five models are listed in Table 4. We can clearly see a big vary between the values of different models, which may be caused by the different influence factors considered by each formula. Because these designed molecules have no specific experimental values for H₅₀, we only compare these values using four models with the experimental values of parent compound. For the experimental value of H₅₀, the parent is 24 cm; for the theoretical value, model 1 is 39.1, model 2 is 126.1 cm, model 3 is 60.98 cm, model 4 is 1.49 cm, and model 5 is 0.13 cm. These results support model 1 is significant in predicting impact sensitivities for our system, and the factors of other models may be minor. So next, we will focus on model 1 because its prediction results are more accurate than those of other models in our system.

  Table 4

  

  Table 4.  Calculated Impact Sensitivity (H₅₀) of the Investigated Molecules and RDX, HMX and CL-20

  DownLoad: CSV

  Compound	$ {\sigma ^2}_ + $(kcal/mol)2	$ \nu $	$ \bar V{s^ + } $(kcal/mol)	$ \bar V{s^ - } $(kcal/mol)	Model 1	Model 2	Model 3	Model 4	Model 5
  Parent	236.38862	0.20550	22.46979	−17.20850	39.1	126.14	60.98	1.49	0.13
  A	235.59966	0.15323	19.70913	−12.94426	27.9	76.61	31.79	4.50	1.81
  B	236.65870	0.19816	21.04538	−16.67048	37.5	171.00	51.44	5.20	3.19
  C	241.85658	0.19262	22.20935	−17.59273	36.3	157.29	46.20	1.47	0.10
  D	282.03397	0.13200	22.98768	−14.25554	23.1	41.99	30.20	4.07	1.32
  E	238.56485	0.16404	20.91799	−14.57266	30.2	87.81	33.50	3.65	1.45
  F	237.46934	0.15127	18.91958	−11.04645	27.5	54.12	31.56	24.61	13.13
  G	260.46121	0.11706	21.08201	−11.83717	20.0	36.38	29.75	189.32	80.31
  H	231.62189	0.19684	17.93323	−13.09988	37.3	145.99	50.06	7.92	5.38
  I	219.31917	0.16648	17.83382	−11.66688	30.9	93.12	34.03	39.85	24.60
  J	259.41534	0.19577	21.71407	−15.74306	36.9	99.37	49.01	1.75	0.34
  K	253.42277	0.15626	19.78583	−12.48135	28.5	64.52	32.18	5.50	2.45
  L	240.46104	0.11492	18.53496	−9.76660	19.7	41.56	29.70	6.03	1.97
  Parenta					24.0
  RDXb					26.0
  HMXb					29.0
  CL-20c					12.0
  a Experimental value from Ref. [7]. b Data from Ref. [22]. c Data from Ref. [23].

  In Table 4, these molecules have H₅₀ values between 19.7 and 37.5 cm. Compound L with C(NO₂)₃ group has the lowest H₅₀, which means it is more sensitive, but compound B with the largest value is more stable. A NH₂ group appears in compound B, which may provide additional hydrogen bond interactions. Thereby, there is a significant increase in H₅₀ in this impact sensitivity. The impact sensitivity H₅₀ of these new molecules was nearly above 26 cm, and most of them were comparable with that of common explosives, RDX (26 cm) and HMX (29 cm)^{[22, 23]}. Besides, the H₅₀ values of all derivatives are higher than CL-20. The sensitivity increased with the number of oxygen atoms in different new groups. The phenomenon may result from oxides that have a strong power to attract electrons, thus reducing their impact sensitivity to some extent. However, owing to the complexity of assessing impact sensitivity, these views could be single rather than conclusive.

  3.4 Detonation performance

  Detonation velocity (D) and detonation pressure (P) are two key explosive parameters for a high energy density material. These parameters can be calculated by the Kamlet Jacobs empirical equations on the basis of the theoretical density (ρ) and heat of detonation (Q) of the energetic materials. The Q and ρ values of synthetic molecules can be measured experimentally, but for some new organic materials, they are very difficult to obtain experimentally. Thus, these two values of designed new compounds have to be shown firstly to gain key parameters. Therefore, the calculated densities (ρ), heats of detonation (Q), detonation velocities (D) and detonation pressures (P) of the designed molecules are shown in Table 5. At the same time, the experimental detonation performances of traditional energetic materials are listed in Table 5 for an intuitive comparison.

  Table 5

  

  Table 5.  Calculated Detonation Properties and Nitrogen Content of the Title Compounds and Reference Compounds TNT, RDX, HMX, FOX-7 and CL-20

  DownLoad: CSV

  Compound	$ \rho 0 $(g/cm3)	$ \rho $(g/cm3)	Q (cal/g)	P (GPa)	D (km/s)	OB100	N%
  Parent		1.86		35.20	8.96	−0.88	49.56
  A	1.92	1.93	1612.93	38.90	9.19	1.11	46.49
  B	1.80	1.88	1583.73	32.35	8.54	−1.24	52.28
  C	1.75	1.83	2083.31	35.57	9.04	−1.56	54.69
  D	1.85	1.86	1634.37	36.98	9.06	0.70	48.95
  E	1.84	1.87	1659.09	37.67	9.16	0.33	51.16
  F	1.91	1.92	1319.04	35.42	8.78	2.11	46.53
  G	1.74	1.74	1011.45	24.28	7.47	−0.37	57.68
  H	1.91	1.97	1505.06	37.20	9.01	1.74	43.90
  I	1.94	1.96	1244.86	35.68	8.77	2.59	44.38
  J	1.81	1.90	1912.46	37.82	9.22	0.00	46.28
  K	1.96	1.98	1572.42	32.39	8.34	−0.36	45.49
  L	1.77	1.76	1554.97	32.06	8.55	2.70	33.63
  TNTa		1.64	1295	19.00	6.95	−3.08	18.50
  RDXa		1.80	1501	34.70	8.75	0.00	37.84
  HMXa		1.90	1498	39.30	9.10	0.00	37.84
  FOX-7a		1.89	1200	34.00	8.87	0.00	37.84
  CL-20a		2.04	1567	44.10	9.38	1.37	38.36
  a Data from Ref. [24].

  From the table, we can see that values of ρ, Q, D and P are from 1.76 g/cm³ (L) to 1.97 g/cm³ (H), from 1011.45 cal/g (G) to 2083.31 cal/g (C), from 7.47 km/s (G) to 9.19 km/s (A), and from 24.28 GPa (G) to 38.9 GPa (A), respectively. By comparing the ρ value of parent and these new title compounds, we found that the density of most of the molecules is much higher than the density of parent. Besides, the ρ value of the parent is close to that of compound E. Further work was focused on improving the detonation performance of the title compounds by introducing energetic groups which resulted in the second-generation of agent defeat weapons. The introduction of NF₂ is a successful strategy, with the molecular density rising from 1.86 g/cm³ (parent) to 1.98 g/cm³ (K), which is better than that of TNT (1.64 g/cm³), RDX (1.80 g/cm³), HMX (1.90 g/cm³), and FOX-7 (1.89 g/cm³)^[24]. As we know, a molecular density close to 2.0 g/cm³ is desirable until now in the field of explosive. Thus, molecule K is glamorous from the viewpoint of density. As expected, the introduction of nitrogen rich groups leads to higher density than these common explosives have. Compounds A (1.93 g/cm³), F (1.92 g/cm³), H (1.97 g/cm³) and I (1.96 g/cm³) also show better density. After evaluating the physicochemical properties of the title compounds, including density and HOF, our attention has been turned to their detonation properties. As shown in Table 4, the calculated detonation velocity and detonation pressure of compound A are 9.19 km/s and 38.9 GPa, respectively, which are much superior to those of TATB (8.11 km/s and 32.4 GPa), TNT (6.95 km/s and 19.00 GPa) and RDX (8.75 km/s and 34.7 GPa)^[24]. The detonation velocity and pressure of all compounds are also superior to those of TNT. But compound G (24.28 GPa) has lower detonation pressure than HMX (39.2 GPa), FOX-7 (35.9 GPa), and RDX (34.9 GPa)^[24].

  Furthermore, oxygen balance (OB) is also an important parameter for energetic materials to determine whether the compounds are oxygen-enriched or oxygen-poor. Here, for a compound with molecular formula C_aH_bO_cN_d, the oxygen balance can be represented as equation (9)^[25]:

  $ OB_{100} = \frac{{100(2n_{\text{O}} - n_{\text{H}} - 2n_{\text{C}} - 2n_{\text{COO}})}}{M} $

  (9)

  Here$ n{\text{O}} $, $ n{\text{H}} $and$ n{\text{C}} $represent the numbers of O, H and C atoms, respectively; $ {n_{{\text{COO}}}} $is the number of _COO^-, and here $ {n_{{\text{COO}}}} = 0 $ for all derivatives; M is the molecular weight. In this work, the OB values of all designed materials are obtained in Table 5. Besides, nitrogen contents of all compounds are listed in Table 5. As can be seen from OB values, the four molecules (compound B, C, G and K) all show oxygen-poor properties, mainly due to the relatively higher carbon and hydrogen contents. And compounds D and E are close to zero-oxygen balance. Compound J with OH group has the zero-oxygen balance. Besides, for energetic propellant fuels, high nitrogen content is advantageous for smokeless combustion. In the work (in Table 5), the nitrogen contents of all derivatives ranged from 33.63% (compound L) to 54.69% (compound C), and the values of most compounds are higher than that of TNT (18.5%), RDX (37.84%), HMX (37.84%), FOX-7 (78.84%) and CL-20 (38.36%)^[24].

  4. CONCLUSION

  A family of new organic molecules of 4-nitro-5-(5-nitro-1, 2, 4-triazol-3-yl)-2H-1, 2, 3-triazolate are designed. The molecular structures, electronic properties, heat of formation (HOF), impact sensitivity (H₅₀), density (ρ), detonation velocity (D) and detonation pressure (P) of all new compounds are completely characterized by theoretical calculation at the B3LYP/6-311G** level of theory. Besides, oxygen balance (OB) and nitrogen content for all compounds are discussed in this paper. It is noteworthy that all title compounds have excellent HOFs and higher D and P values than TNT. Eight of them (A, C, D, E, F, H, I, J) have higher P values than RDX (34.70 GPa) and FOX-7 (34.00 GPa), and three of them (A, E, J) have higher D values than HMX (9.10 km/s). The good detonation performances of these compounds are caused by outstanding density. Surprisingly, more than half of these derivatives have higher density over 1.80 g/cm³.

  Based on the above results, we can see that new molecule J (H₅₀ = 36.9 cm, ρ = 1.90 g/cm³, Q = 1912.46 cal/g, P = 37.82 GPa, D = 9.22 km/s, OB = 0.00), compound A (H₅₀ = 27.9 cm, ρ = 1.93 g/cm³, Q = 1612.93 cal/g, P = 38.90 GPa, D = 9.19 km/s) and compound H (H₅₀ = 37.3 cm, ρ = 1.97 g/cm³, Q = 1505.06 cal/g, P = 37.20 GPa, D = 9.01 km/s) can be considered as potential candidates in terms of the energetic material. Especially for compound J, the good balance of detonation performance and sensitivity, plus the environmental oxygen balance, contribute to its practical application as a promising primary explosive. It is a green and powerful alternative to the toxic and sensitive explosives.

  
  
• 1. [1]

     Tang, Y. X.; Huang, W.; Chinnam, A. K.; Singh, J. S.; Staples, R. J.; Shreeve, J. M. Energetic tricyclic polynitropyrazole and its salts: proton-locking effect of guanidium cations. Inorg. Chem. 2021, 60, 8339−8345. doi: 10.1021/acs.inorgchem.1c01202

  2. [2]

     Zhang, W. Q.; Zhang, J. H.; Deng, M. C.; Qi, X. J.; Nie, F. D.; Zhang, Q. H. A promising high-energy-density material. Nat. Commun. 2017, 8, 181−187. doi: 10.1038/s41467-017-00286-0

  3. [3]

     Eberly, J. O.; Mayo, M. L.; Carr, M. R.; Crocker, F. H.; Indest, K. J. Detection of hexahydro-1, 3-5-trinitro-1, 3, 5-triazine (RDX) with a microbial sensor. J. Gen. Appl. Microbiol. 2019, 64, 139−144.

  4. [4]

     He, P.; Zhang, J. G.; Wang, K.; Yin, X.; Jin, X.; Zhang, J. G. Extensive theoretical studies on two new members of the FOX-7 family: 5-(dinitromethylene)-1, 4-dinitramino-tetrazole and 1, 1΄-dinitro-4, 4΄-diamino-5, 5′-bitetrazole as energetic compounds. Phys. Chem. Chem. Phys. 2015, 17, 5840−5848. doi: 10.1039/C4CP04883K

  5. [5]

     Ariyarathna, T.; Ballentine, M.; Vlahos, P.; Smith, R. W.; Cooper, C.; Bohlke, J. K.; Fallis, S.; Groshens, T. J.; Tobias, C. Tracing the cycling and fate of the munition, hexahydro-1, 3, 5-trinitro-1, 3, 5-triazine in a simulated sandy coastal marine habitat with a stable isotopic tracer, (15)N-[RDX]. Sci. Total Environ. 2019, 647, 369−378. doi: 10.1016/j.scitotenv.2018.07.404

  6. [6]

     Tang, Y. X.; He, C. L.; Imler, G. H.; Parrish, D. A.; Shreeve, J. M. Aminonitro groups surrounding a fused pyrazolotriazine ring: a superior thermally stable and insensitive energetic material. ACS Appl. Energy Mater. 2019, 2, 2263−2267. doi: 10.1021/acsaem.9b00049

  7. [7]

     Xu, Z.; Cheng, G. B.; Zhu, S. G.; Lin, Q. H.; Yang, H. W. Nitrogen-rich salts based on the combination of 1, 2, 4-triazole and 1, 2, 3-triazole rings: a facile strategy for fine tuning energetic properties. J. Mater. Chem. A 2018, 6, 2239–2248. doi: 10.1039/C7TA08941D

  8. [8]

     Hehre, W. J.; Ditchfield, D.; Radom, L.; Pople, J. A. Molecular orbital theory of the electronic structure of organic compounds. V. Molecular theory of bond separation. J. Am. Chem. Soc. 1970, 92, 4796–4801. doi: 10.1021/ja00719a006

  9. [9]

     Zhang, J. C.; Zhang, J. H.; Imler, G. H.; Parrish, D. A.; Shreeve, J. M. Sodium and potassium 3, 5-dinitro-4-hydropyrazolate: three dimensional metal-organic frameworks as promising super-heatresistant explosives. ACS Appl. Energy Mater. 2019, 2, 7628–7634. doi: 10.1021/acsaem.9b01608

  10. [10]

      Wang, Q.; Shao, Y. L.; Lu, M. C₈N₁₂O₈: a promising insensitive high-energy-density material. Cryst. Growth Des. 2018, 18, 6150–6154. doi: 10.1021/acs.cgd.8b01016

  11. [11]

      Li, B. T.; Li, L. L.; Peng, J. Theoretical exploration about the detonation performance and thermal stability of the nitro-substituted derivatives of guanine. Chin. J. Struct. Chem. 2021, 40, 409–414.

  12. [12]

      Frisch, M. J.; Trucks, G. W.; Schlegel, H. B.; Scuseria, G. E.; Robb, M. A.; Cheeseman, J. R.; Scalmani, G.; Barone, V.; Mennucci, B. G.; Petersson, A.; Nakatsuji, H.; Caricato, M.; Li, X.; Hratchian, H. P.; Izmaylov, A. F.; Bloino, J.; Zheng, G.; Sonnenberg, J. L.; Hada, M.; Ehara, M.; Toyota, K.; Fukuda, R.; Hasegawa, J.; Ishida, M.; Nakajima, T.; Honda, Y.; Kitao, O.; Nakai, H.; Vreven, T.; Montgomery Jr. J. A.; Peralta, J. E.; Ogliaro, F.; Bearpark, M.; Heyd, J. J.; Brothers, E.; Kudin, K. N.; Staroverov, V. N.; Kobayashi, R.; Normand, J.; Raghavachari, K.; Rendell, A.; Burant, J. C.; Iyengar, S. S.; Tomasi, J.; Cossi, M.; Rega, N.; Millam, J. M.; Klene, M.; Knox, J. E.; Cross, J. B.; Bakken, V.; Adamo, C.; Jaramillo, J.; Gomperts, R.; Stratmann, R. E.; Yazyev, O.; Austin, A. J.; Cammi, R.; Pomelli, C.; Ochterski, J. W.; Martin, R. L.; Morokuma, K.; Zakrzewski, V. G.; Voth, G. A.; Salvador, P.; Dannenberg, J. J.; Dapprich, S.; Daniels, A. D.; Farkas, O.; Foresman, J. B.; Ortiz, J. V.; Cioslowski, J.; Fox, D. J. Gaussian 09, Revision D. 01, Gaussian, Inc., Wallingford CT 2009.

  13. [13]

      He, P.; Zhang, J. G.; Wang, K.; Yin, X.; Zhang, J. G. Computational studies on two novel energetic nitrogen-rich compounds based on tetrazolone. J. Phys. Org. Chem. 2015, 3484–3489.

  14. [14]

      Politzer, P.; Lane, P.; Murray, J. S. Computational characterization of a potential energetic compound: 1, 3, 5, 7-tetranitro-2, 4, 6, 8-tetraazacubane. Cent. Eur. J. Energ. Mater. 2011, 8, 39–52.

  15. [15]

      Rice, B. M.; Pai, S. V.; Hare, J. Predicting heats of formation of energetic materials using quantum mechanical calculations. Combust. Flame. 1999, 118, 445–458. doi: 10.1016/S0010-2180(99)00008-5

  16. [16]

      Kamlet, M. J.; Jacobs, S. J. Chemistry of detonations. I. A simple method for calculating detonation properties of C–H–N–O explosives. J. Chem. Phys. 1968, 48, 23–35. doi: 10.1063/1.1667908

  17. [17]

      Politzer, P.; Murray, J. S. The fundamental nature and role of the electrostatic potential in atoms and molecules. Theor. Chem. Acc. 2002, 108, 134–142. doi: 10.1007/s00214-002-0363-9

  18. [18]

      Pospíšil, M.; Vávra, P.; Concha, M. C.; Murray, J. S.; Politzer, P. A possible crystal volume factor in the impact sensitivities of some energetic compounds. J. Mol. Model. 2010, 16, 895–901. doi: 10.1007/s00894-009-0587-x

  19. [19]

      Rice, B. M.; Hare, J. J. A quantum mechanical investigation of the relation between impact sensitivity and the charge distribution in energetic molecules. J. Phys. Chem. A 2002, 106, 1770–1783. doi: 10.1021/jp012602q

  20. [20]

      Christe, K. O.; Wilson, W. W.; Sheehy, J. A.; Boatz, J. A. N⁵⁺: a novel homoleptic polynitrogen ion as a high energy density material. Angew. Chem. Int. Ed. 1999, 38, 2004–2009. doi: 10.1002/(SICI)1521-3773(19990712)38:13/14<2004::AID-ANIE2004>3.0.CO;2-7

  21. [21]

      Song, X. L.; Li, J. C.; Hou, H.; Wang, B. S. Extensive theoretical studies of a new energetic material: tetrazino-tetrazine-tetraoxide (TTTO). J. Comput. Chem. 2009, 30, 1816–1820. doi: 10.1002/jcc.21182

  22. [22]

      Guo, C.; Zhang, H.; Wang, X.; Liu, X.; Sun, J. Study on a novel energetic cocrystal of TNT/TNB. J. Mater. Sci. 2013, 48, 1351–135. doi: 10.1007/s10853-012-6881-5

  23. [23]

      Li, B. T.; Li, L. L.; He, J. X. Looking for high energy density molecules in the nitro-substituted derivatives of pyridazine. Chin. J. Struct. Chem. 2020, 39, 849–854

  24. [24]

      Politzer, P.; Murray, J. S. Some perspectives on estimating detonation properties of C, H, N and O compounds. Cent. Eur. J. Energ. Mater. 2011, 8, 209–220.

  25. [25]

      Zhang, C.; Shu, Y.; Huang, Y.; Zhao, X.; Dong, H. Investigation of correlation between impact sensitivities and nitro group charges in nitro compounds. J. Phys. Chem. B 2005, 109, 8978–8982. doi: 10.1021/jp0512309

• 

  Figure 1  All molecular structures of the title derivatives

  下载: 全尺寸图片 幻灯片

  Table 1.  Calculated Methods for the Values of N, $ \bar M $, and Q of Explosive C_aH_bO_cN_d. $ \bar M $ is the Molecular Weight in g/mol and$ \Delta H{f^0} $the Solid Phase HOF in kcal/mol

  Parameters	Explosives components conditions
  	c≥2a + b/2	2a + b/2 > c≥b/2	b/2 > c
  $ N $	(b + 2c + 2d)/4M	(b + 2c + 2d)/4M	(b + d)/2M
  $ \bar M $	4M/(b + 2c + 2d)	(56d + 88c-8b)/(b + 2c + 2d)	(2b + 28d + 32c)/(b + d)
  Q×10-3	(28.9b + 94.05a + 0.239$ {\Delta}H{f^0} $)/M	[28.9b + 94.05(c/2-b/4) + 0.239$ {\Delta}H{f^0} $]/M	(57.8c + 0.239$ {\Delta}H{f^0} $)/M

  下载: 导出CSV

  Table 2.  Calculated Total Energies (E₀, a.u.), Zero-point Energies (ZPE, a. u.), Thermal Corrections (H_T, a. u.), and Solid Phase Heats of Formation (HOFs, Solid, kJ/mol) of Twelve New High Explosives Compared to Traditional Explosives

  Compound	E0 (a.u.)	ZPE (a.u.)	HT (a.u.)	HOFs (kJ/mol)
  Parent				559.59
  A	−1096.99510	0.10545	0.01582	560.54
  B	−947.83270	0.12144	0.01438	636.49
  C	−1003.16217	0.13797	0.01572	727.30
  D	−1152.35009	0.12294	0.01681	585.48
  E	−1207.69207	0.13888	0.01832	639.04
  F	−1356.85629	0.12292	0.01980	551.73
  G	−1055.91629	0.10359	0.01623	1341.05
  H	−1172.18564	0.10926	0.01682	470.28
  I	−1432.04593	0.12635	0.02093	470.54
  J	−967.67723	0.10831	0.01396	504.66
  K	−1146.21206	0.10244	0.01606	533.47
  L	−1545.38885	0.13719	0.02230	326.75
  TNTa				−63.12
  RDXa				79.00
  HMXa				75.24
  FOX-7b				−133.70
  CL-20a				377.04
  a Data from Ref. [21]. b Data from Ref. [15].

  下载: 导出CSV

  Table 3.  Frontier Orbital Energies and Their Differences of Title Compounds at the B3LYP/6-311G** Level of Theory

  Compound	HOMO	LUMO	$ \Delta ELUMO - HOMO $
  Parent	−0.3036	−0.13928	0.1644
  A	−0.3369	−0.1441	0.1929
  B	−0.3027	−0.1266	0.1761
  C	−0.3192	−0.1160	0.2032
  D	−0.3228	−0.1278	0.1950
  E	−0.3094	−0.1226	0.1868
  F	−0.3360	−0.1385	0.1975
  G	−0.3257	−0.1602	0.1655
  H	−0.3128	−0.13441	0.1784
  I	−0.3223	−0.1313	0.1910
  J	−0.3053	−0.1465	0.1589
  K	−0.3371	−0.1325	0.2046
  L	−0.3438	−0.1577	0.1860

  下载: 导出CSV

  Table 4.  Calculated Impact Sensitivity (H₅₀) of the Investigated Molecules and RDX, HMX and CL-20

  Compound	$ {\sigma ^2}_ + $(kcal/mol)2	$ \nu $	$ \bar V{s^ + } $(kcal/mol)	$ \bar V{s^ - } $(kcal/mol)	Model 1	Model 2	Model 3	Model 4	Model 5
  Parent	236.38862	0.20550	22.46979	−17.20850	39.1	126.14	60.98	1.49	0.13
  A	235.59966	0.15323	19.70913	−12.94426	27.9	76.61	31.79	4.50	1.81
  B	236.65870	0.19816	21.04538	−16.67048	37.5	171.00	51.44	5.20	3.19
  C	241.85658	0.19262	22.20935	−17.59273	36.3	157.29	46.20	1.47	0.10
  D	282.03397	0.13200	22.98768	−14.25554	23.1	41.99	30.20	4.07	1.32
  E	238.56485	0.16404	20.91799	−14.57266	30.2	87.81	33.50	3.65	1.45
  F	237.46934	0.15127	18.91958	−11.04645	27.5	54.12	31.56	24.61	13.13
  G	260.46121	0.11706	21.08201	−11.83717	20.0	36.38	29.75	189.32	80.31
  H	231.62189	0.19684	17.93323	−13.09988	37.3	145.99	50.06	7.92	5.38
  I	219.31917	0.16648	17.83382	−11.66688	30.9	93.12	34.03	39.85	24.60
  J	259.41534	0.19577	21.71407	−15.74306	36.9	99.37	49.01	1.75	0.34
  K	253.42277	0.15626	19.78583	−12.48135	28.5	64.52	32.18	5.50	2.45
  L	240.46104	0.11492	18.53496	−9.76660	19.7	41.56	29.70	6.03	1.97
  Parenta					24.0
  RDXb					26.0
  HMXb					29.0
  CL-20c					12.0
  a Experimental value from Ref. [7]. b Data from Ref. [22]. c Data from Ref. [23].

  下载: 导出CSV

  Table 5.  Calculated Detonation Properties and Nitrogen Content of the Title Compounds and Reference Compounds TNT, RDX, HMX, FOX-7 and CL-20

  Compound	$ \rho 0 $(g/cm3)	$ \rho $(g/cm3)	Q (cal/g)	P (GPa)	D (km/s)	OB100	N%
  Parent		1.86		35.20	8.96	−0.88	49.56
  A	1.92	1.93	1612.93	38.90	9.19	1.11	46.49
  B	1.80	1.88	1583.73	32.35	8.54	−1.24	52.28
  C	1.75	1.83	2083.31	35.57	9.04	−1.56	54.69
  D	1.85	1.86	1634.37	36.98	9.06	0.70	48.95
  E	1.84	1.87	1659.09	37.67	9.16	0.33	51.16
  F	1.91	1.92	1319.04	35.42	8.78	2.11	46.53
  G	1.74	1.74	1011.45	24.28	7.47	−0.37	57.68
  H	1.91	1.97	1505.06	37.20	9.01	1.74	43.90
  I	1.94	1.96	1244.86	35.68	8.77	2.59	44.38
  J	1.81	1.90	1912.46	37.82	9.22	0.00	46.28
  K	1.96	1.98	1572.42	32.39	8.34	−0.36	45.49
  L	1.77	1.76	1554.97	32.06	8.55	2.70	33.63
  TNTa		1.64	1295	19.00	6.95	−3.08	18.50
  RDXa		1.80	1501	34.70	8.75	0.00	37.84
  HMXa		1.90	1498	39.30	9.10	0.00	37.84
  FOX-7a		1.89	1200	34.00	8.87	0.00	37.84
  CL-20a		2.04	1567	44.10	9.38	1.37	38.36
  a Data from Ref. [24].

  下载: 导出CSV
•