English

• Electrospun fiber mats (EFM) made of functional molecules and biocompatible polymers have been widely used in wound dressing [1, 2], tissue engineer [3-5], anticancer [6], anti-oxidant and antibacterial coatings [7]. The morphology of EFM has strong influence on its bioactivities and mechanics [1, 8, 9]. According to our recent work [10], the morphology of EFM made up of zein and PEO (polyethylene oxide) can be well regulated through the concentration and composition, viscosity and surface tension of solutions. While the clear correlation between solution structure and EFM morphology is still not clear yet.

  Zein is an ever widely used natural material with versatile applications [11-13], such as in drug delivery, encapsulation, functional fiber, adhesive, coating, ceramic, ink, cosmetic, textile, chewing gum and so on. It received intensive studies in recent two decades as candidates for biocompatible and environmental friendly materials, especially under the scenario of electrospinning [14, 15]. The major sub-type of zein is α-zein, which contains two fractions, named as Z19 and Z22 with molecular weight around 19 kDa and 22 kDa [16], respectively. The sequence and 3D structure of zein still varied in a range, and a number of structural models with high-degree consensus in secondary structure and folding have been proposed [17]. Matsushima et al. proposed an elongated model with an axial ratio of 6:1 based on small angle Xray scattering (SAXS) characterization, the fold contains single α-helix tandem anti-parallel repeat units and glutamine-rich 'turns' or loops [18]. Forato et al. constructed a hairpin model with the axial ratio spans from 3:1 to 6.5:1, made up by extended helix, sheet and turns based on FTIR, SAXS and NMR characterizations [19]. A 3D structure model with assembled 9 helical segments into 3 interacted coiled-coil helices built using molecular mechanics and dynamics simulations was also reported [20]. Through an integration of SAXS, structure prediction, simulation and rheology, we addressed the 3D structure and its dispersion status in variant solvents, including acetic acid, acidic aqueous and ethanol aqueous solutions [16, 21]. Recently, Uzun et al. found the aging time has significant impact on the structure, the aggregation and the rheology of zein in ethanol aqueous solutions [22]. Polymer like PEO is flexible to interact with proteins and facilitate the formation of fibers [23], and alternative polymers [24] with similar properties have been widely integrated to enhance electrospinnability. Our recent findings revealed that the solution properties of zein and PEO have strong correlation with EFM [10]. To unveil the structure of solutions and construct their correlation relationship with EFM and rheology behaviors thus should shed insight to understand the interplay of protein and polymer during the solidification in electrospinning.

  In this work, SAXS, rheology and electrospinning were performed to study zein and PEO in aqueous ethanol solutions, attempt to understand the solution and the resulted EFM from the structure and the relaxation of structures. Materials and methods will be presented in the next section, followed by the study on the structure and rheology of solutions, the aging effect of zein and representative morphologies for EFMs.

  Zein (Lots: LS50O92, J & K Scientific Ltd.) and PEO (Lots: T28B045, Alfa Aesar Ltd.) were dissolved in 80% (v/v) aqueous ethanol at room temperature. The average molecular weight for zein is 19~22 kDa and PEO is 1000 kDa. Ethanol is AR grade, distilled water and solvent with 80% ethanol and water (v/v) were used in all experiments. The aging samples were stored at room temperature for certain time before electrospun or SAXS characterization.

  The SAXS experiments were carried out at the BL19U2 beamline of Shanghai Synchrotron Radiation Facility (SSRF). The beam is at 12 keV and the energy resolution of 5×10^-4. The wavelength of Xray radiation is 1.033 Å and spot of 380×110 μm². The sample to detector distance is 2180 mm with Pilatus 1 M detector. The scattering vector Q ranged from 5.0×10^-3 Å^-1 to 0.4 Å^-1. Samples were enwrapped in a polymethyl methacrylate cell in 3 mm in thickness, and sealed by polyimide tapes on the path of X-ray beam. The explorer time is 1 s, and 5 randomly located spots were collected for each sample. The final SAXS scattering intensity profiles were obtained through the average of 5–15 convergent curves followed by the subtraction of buffer and cell background.

  Solution rheology characterizations were performed on AntonPaar MC302 rheometer, with temperature controlled at 25±0.2℃. Samples of 0.6~0.8 mL were loaded under a 50 mm cone plate, sealed with paroline at the edge, and a 0.099 mm gap were applied at the shear rate from 0.1 s^-1 to 1000 s^-1. Oscillatory time sweep at 1 Hz and 0.3% strain for 25 wt% zein solutions.

  A horizontally settled electrospinning apparatus was used to prepare EFMs. The high direct voltage is fixed at 15 kV between the syringe and collector. The syringe is connected with a stainless steel needle with 0.51 mm internal diameter and fixed flux of solutions at 2 mL/h. A separation distance of 11 cm was held between the needle tip and the surface of collector, and the collector was rolled at 1000 rpm, followed the procedure similar to our recent work [10]. The temperature and the relative humidity were controlled at 25±2℃ and (50±5)% for all experiments. Air and bubbles were careful expelled before electrospinning.

  The SAXS intensity profiles and the corresponding DebyeBueche plots of zein in 80% aqueous ethanol solutions are presented in Figs. 1a and b. The scattering intensity increases with concentration at small Q range (Q < 0.04 Å^-1) that reveals the formation of finite-sized aggregates. The fractal dimension at this range increases from 2.36 to 3.61, suggesting the aggregates have Gaussian distributed loosely packed network at low concentration, and compacted aggregates with rough interface at high concentration. While at large Q range, the fractal dimension decreases from 1.66 to 1.21 with concentration increases. It agrees with our previous observations [21] for the rod-like conformation of proteins, increasing concentration may promote intermolecular helix formation as proposed by Matsushima et al. [18], and the rodlike growth of zein aggregates suggested by Uzun et al. [22] that cause the fractal dimension approaches to 1. The solid lines are the Guinier fittings that provide the gyration radii (R_g) for individual proteins, which are summarized in Table 1. The Rg slightly decreases with concentration, analogue to the crowding and screening induced collapse in typical polymer solutions [25]. The correlation lengths (ξ), i.e., the size of zein aggregates can be extracted from the Debye-Beuche plot. It increases with concentration, indicates the growth of zein aggregates. Then the aggregation number, i.e., the average number of proteins in an aggregate (i.e., protein oligomer), can be estimated through (ξ/R_g)³. It spans from 2.5 to 55.2 at the studied concentration range, further validated the formation of finite-sized aggregates.

  Figure 1

  

  Figure 1.  SAXS intensity profiles (a) and the corresponding Debye-Bueche plots (b) of zein in 80% aqueous ethanol solutions. The profiles (c) and the corresponding pair distance distribution function (d) of PEO in 80% aqueous ethanol solutions. The profiles of zein-PEO mixed solutions (e) with fixed total concentration of 16 wt% at different fractions of zein, and the schematic plot for the structure change against the fraction of zein, aging time and total concentration (f). Lines in (a, b, c, e) are the best fitting to either a fractal zone or the original experimental points. Ellipsoid model is used to fit SAXS signal of zein in (a), and Debye model [28] is used to fit SAXS signal of PEO in (c). Experimental errors are less than the size of symbol so error bars are ignored.

  DownLoad: Full-Size Img PowerPoint

  Table 1

  

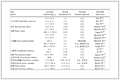  Table 1.  Structure and dynamic parameters derived from SAXS and rheology experiments for zein and PEO in aqueous ethanol solutions. The fraction of zein was fixed at 0.5 for the mixed solutions.

  DownLoad: CSV

  Our recentworkfound that thefresh zein solutionhasNewtonian rheological behavior, and the viscosity increases with the concentration following typical scaling relationship like neutral polymer solutions [10]. Such rheology behavior and relaxation dynamics should be understood based on the change of solution structures. Typically, the relocation of molecules or aggregates in solutions can be described in terms of the free path and the collision of other domains. Thus, through the combination of the Stokes-Einstein relation R=kT/6πη₀D and the length of free path R² = 6Dτ, we can solve three parameters: the diffusion coefficient D, the size of a domain R and the corresponding relaxation time τ. Here η₀ is the zero-shear viscosity of the solution, k and T are the Boltzmann constant and absolute temperature, respectively. The R can be R_sd or R_avg, theyare defined as R_sd = (0.75πV)^1/3 and R_avg= (75M/πρCN_A)^1/3. Here V is the scattering volume calculated from SAXS intensity profiles using Porod integration [26], M is the molecular weight, ρ is the density of solution, C is weight concentration and N_A is the Avogadro's number. R_sd represents the length of free path by the self-diffusion of a particle, and R_avg is the half of the average separation distance assuming molecules and aggregates are evenly dispersed in the solution. The actual size for zein in solution R_sd is smaller than the R_avg, and the difference becomes smaller with concentration increase. Since R_sd is contributed by zein and the hydration layer, increasing zein concentration results in the hydration of more solvents, the solutions become more homogeneous at high concentrations. The relaxation time for the relocation of zein or zein aggregation in solutions τ_sd is at the same magnitude as that with evenly molecular dispersion, and the actual value reveals from the combination of rheology and SAXS is about half the time of τ_avg. These results clear show that zein formed finite-sized oligomers, and these oligomers are evenly distributed in the solutions. Further, the diffusion coefficient of particles in solutions were calculated using D = kT/6πη₀R_sd. Accordingly, τ_sd and τ_avg were calculated through τ_sd = R_sd²/6D and τ_avg = R_avg²/6D, respectively. According to the Einstein-Stokes equation, where D×R is a constant in identical continuous phase, and the diffusion coefficient for individual water molecule with 2.8 Å in diameter and the selfdiffusion of water at 298 K is 2.3×10^-5 cm²/s [27], we can estimate the diffusion coefficients of zein and PEO. They are 1.7×10^-6 cm²/s and 4.4×10^-7 cm²/s by taking their R_g at the lowest concentration. The actual diffusion coefficients for zein and PEO in the solutions are 1 to 2 magnitudes lower than the estimation. It suggests both polymers are strongly hydrated in the solution and the hydration radius may be significantly larger than the gyration radius. Further, the correlation length, annotated as the size of zein aggregates increases with concentration, but the radius of average space occupied by single zein molecule decreased, this indicates that zein formed prominent aggregation in relatively high concentration.

  Figs. 1c and d show the scattering intensity profiles and the corresponding pair distance distribution functions (PDDF) for PEO solutions. They behaves like typical linear polymers in theta solvent, as revealed from the fractal dimension at small Q region, which is around 2, an indication of Gaussian chain. At large Q region, the fractal dimension is between 1.2 and 1.7, which suggests that ethanol and water may have different affinity to distribute along PEO chains, that make the hydrated polymer blob behaves like rigid chains at local region. At the whole Q range, the scattering intensity profiles for PEO solutions can be well fitted by GNOM [29] and present a quite convergent R_g. The shape of PDDF again, indicates that the conformation of PEO is Gaussian like with slightly ellipsoidal distribution [30], and the longest eigenvalue may be along the end-to-end vector [31].

  The scattering intensity profiles for zein/PEO mixed solutions are shown in Fig. 1e. The upturn of the scattering intensity at very small Q range is flattened by the fractal structure of PEO, which confirmed that proteins are distributed along polymer chains at large spatial scale. Chodankar et al. [32] has also reported such distribution of proteins along polyelectrolyte chains, where bovine serum albumin was found to distribute along sodium polystyrene sulfonate chains. At intermediate Q range, the clear domain interface for zein aggregates was also screened out by the loosely packed PEO chains.

  Fig. 2 presents the intensity profiles and the corresponding Holtzer plot for freshly prepared (within 2.5 h) and aged (incubated at room condition for 4 days) zein solutions. The results agree with a recent SAXS report by Uzun et al. [22]. Moreover, we found a characteristic size of 239.1 Å which is an indication of rod-like aggregates, disappeared in aged solutions. Most probably is that aging promoting protein unfolding and aggregation, which results in larger aggregates and the peak in Holtzer plot shift to smaller Q range that locates out of current experimental window.

  Figure 2

  

  Figure 2.  SAXS intensity profiles (a) and Holtzer plots (b) for 120 mg/mL zein solutions at aging time of 2.5 h and 4 days.

  DownLoad: Full-Size Img PowerPoint

  According to our previous study, aging effect is significant only for solutions at high concentrations [21]. Two concentrated solutions are studied and their structure parameters are listed in Table 2. The correlation length and R_g did not show obvious change after aging, but the R_sd remarkably increased, especially in the 200 mg/mL solution. It shows that the folded domain of zein almost kept unchanged, while the hydration was greatly promoted during aging.

  Table 2

  

  Table 2.  Structure parameters extracted from SAXS for fresh and aged zein solutions.

  DownLoad: CSV

  To further investigate the aging effect, solution viscosity at different aging time is presented in Fig. 3a. The fresh solution behaves like typical Newtonian fluid, shear thinning was promoted with longer aging time. The viscosity approaching to zero-shear rate increases around one magnitude, which is a direct proof for the presence of large aggregates, as suggested from the SAXS characterization. An oscillation time sweep for the fresh zein solution was performed and the storage (G') and loss moduli (G"), as well as the complex viscosity against time are shown in Fig. 3b. The solution translates from viscous to viscoelastic fluid, analogous to a sol-gel transition and the crossover time is around 1.54 h. Such transition is a result from the multi-scale spatial-temporal interplay of zein-rich domains and their relaxation. Further, the complex viscosity of the solution monotonously increases with aging time, consistent with the increasing of shear thinning behavior.

  Figure 3

  

  Figure 3.  Viscosity of zein in 80% (v/v) aqueous ethanol solutions at different aging time (a), and oscillatory time sweep at 1 Hz and 0.3% strain for 25 wt% zein solutions (b).

  DownLoad: Full-Size Img PowerPoint

  As a function of total concentration, the fraction of zein and aging time, at an optimal electrospinning condition, the morphologies of EFMs are presented in Fig. 4. At high concentration and aged solutions, typical ribbon morphology can be observed [33]. Increasing the total concentration gets better fiber mats with less beads or thicker fibers because of higher viscosity [10]. While increase the fraction of zein leads to more beads in fibers, or get ribbons rather than fibers at longer aging time. These impacts are also consistent with the changes in microstructures of solutions as revealed from Fig. 1 and Table 1. Increase total concentration leads larger and denser-distributed aggregates, which enables the formation of continuous fibers, or thickening fibers to be ribbon. Increase f(zein) can coarsen aggregates and concomitantly decrease the volume fraction of polymer-rich domains (because the interior of zein is unhydrated in fresh solution), which increase beads at lower concentration boundary and the thickness of fibers at higher concentration boundary. The longer aging time makes zein-rich oligomers more hydrated without decrease the volume fraction of polymer-rich domains, facilitate the formation of beadfree fibers at low concentration and ribbons at high concentration. Considering the phase diagram for electrospun fibers in our recent work [10], it is relatively easy to provide concentration, f(zein), viscosity and surface tension boundary for fine fibers. For the microstructure of solutions, a τ_sd longer than around 10^-5 s is probably correlated with the formation of continuous fibers or ribbons. It is applicable for zein, PEO and zein/PEO mixed solutions.

  Figure 4

  

  Figure 4.  Morphologies of electrospun products captured by SEM at different concentrations, fractions of zein and aging time, and the total concentrations were labeled. Images a→b & a'→b', f(zein) from 0.3 to 0.9 from fresh solutions; images c→d & c'→d', aging time from fresh to 5 days with f(zein) of 1.0.

  DownLoad: Full-Size Img PowerPoint

  Solution physical properties are the prerequisite of certain EFM morphology, appropriate viscosity ensure the formation of fiber not beads; the hydration of hydrophobic zein determines the quantity of beads and thickness of fiber. Viscosity is adjusted via total concentration and f(zein), and hydration of zein is adjusted via aging time.

  In this study, the solutions of zein, PEO or their mixture in 80% ethanol water were studied by SAXS and rheology. The correlation between the structure, structure relaxation with the morphologies of electrospun products were explored as a function of total concentration, the fraction of zein and aging time. Their impacts are consistent in the structure changes, the relaxation of structure domains and the morphologies of electrospun products in beads, fibers and ribbons. Zein solutions exhibit a viscous to viscoelastic transition at oscillatory time of 1.54 h, together with the enhancement of shear thinning at longer aging time. Increasing the volume fraction of polymer-rich domains through the increase of total concentration and aging time both can facilitate the formation of continuous fibers or ribbons.

  Acknowledgments

  This work was supported by the National Natural Science Foundation of China (Nos. 21374117 and 21774128), Major State Basic Research Development Program (No. 2015CB655302), Key Research Program of Frontier Sciences (No. QYZDY-SSW-SLH027) and One Hundred Person Project of the Chinese Academy of Sciences. We thank the staffs from BL19U2 beamline of National Center for Protein Sciences Shanghai (NCPSS) for SAXS facility support.

• 1. [1]

     A.R. Unnithan, G. Gnanasekaran, Y. Sathishkumar, Y.S. Lee, C.S. Kim, Carbohydr. Polym. 102(2014) 884-892. doi: 10.1016/j.carbpol.2013.10.070

  2. [2]

     Z.W. Li, C.W. Li, Q. Wang, et al., J. Biomed. Nanotechnol. 13(2017) 17-34. doi: 10.1166/jbn.2017.2324

  3. [3]

     S.K. Nitta, K. Numata, Int. J. Mol. Sci. 14(2013) 1629-1654. doi: 10.3390/ijms14011629

  4. [4]

     Y. Qu, B. Wang, B. Chu, et al., ACS Appl. Mat. Interfaces 10(2018) 4462-4470. doi: 10.1021/acsami.7b17020

  5. [5]

     J. Amirian, S.Y. Lee, B.T. Lee, J. Biomed. Nanotechnol. 12(2016) 1864-1875. doi: 10.1166/jbn.2016.2308

  6. [6]

     S. Iqbal, M.H. Rashid, A.S. Arbab, M. Khan, J. Biomed. Nanotechnol. 13(2017) 355-366. doi: 10.1166/jbn.2017.2353

  7. [7]

     Y.H. Tsai, Y.N. Yang, Y.C. Ho, M.L. Tsai, F.L. Mi, Carbohydr. Polym. 180(2018) 286-296. doi: 10.1016/j.carbpol.2017.09.100

  8. [8]

     J. Li, Towards Biopolymer Platforms via Small Molecule Crosslinking, Organocatalytic Ring-opening Polymerization and Electrospinning, PhD Thesis, Rutgers, The State University of New Jersey, New Brunswick, NJ, 2013.

  9. [9]

     X. Li, J. Wang, Z. Hu, M. Li, K. Ogino, Chin. Chem. Lett. 29(2018) 166-170. doi: 10.1016/j.cclet.2017.05.020

  10. [10]

      H. Zhang, S. Xi, Y. Han, et al., Soft Matter 14(2018) 3455-3462. doi: 10.1039/C7SM02203D

  11. [11]

      R. Shukla, M. Cheryan, Ind. Crops Prod. 13(2001) 171-192. doi: 10.1016/S0926-6690(00)00064-9

  12. [12]

      J.W. Lawton, Cereal Chem. 79(2002) 1-18. doi: 10.1094/CCHEM.2002.79.1.1

  13. [13]

      M.M. Algandaby, M.M. Al-Sawahli, O.A.A. Ahmed, et al., J. Biomed. Nanotechnol. 12(2016) 1746-1757. doi: 10.1166/jbn.2016.2270

  14. [14]

      S.B. Yang, S.H. Yoo, M.M. Rabbani, et al., J. Nanosci. Nanotechnol. 17(2017) 9002-9008. doi: 10.1166/jnn.2017.14370

  15. [15]

      L.L. Deng, X. Zhang, Y. Li, et al., Food Hydrocoll. 75(2018) 72-80. doi: 10.1016/j.foodhyd.2017.09.011

  16. [16]

      Y. Li, Q. Xia, K. Shi, Q. Huang, J. Phys. Chem. B 115(2011) 9695-9702. http://cn.bing.com/academic/profile?id=3b19457c7c7a6771a182a8afbc8fc452&encoded=0&v=paper_preview&mkt=zh-cn

  17. [17]

      H. Turasan, J.L. Kokini, Biomacromolecules 18(2017) 331-354. doi: 10.1021/acs.biomac.6b01455

  18. [18]

      N. Matsushima, G.I. Danno, H. Takezawa, Y. Izumi, Biochim. Biophys. Acta 1339(1997) 14-22. doi: 10.1016/S0167-4838(96)00212-9

  19. [19]

      L.A. Forato, A.C. Doriguetto, H. Fischer, et al., J. Agric. Food Chem. 52(2004) 2382-2385. doi: 10.1021/jf035020+

  20. [20]

      F.A. Momany, D.J. Sessa, J.W. Lawton, et al., J. Agric. Food Chem. 54(2006) 543-547. doi: 10.1021/jf058135h

  21. [21]

      Y. Li, J. Li, Q. Xia, et al., J. Phys. Chem. B 116(2012) 12057-12064. doi: 10.1021/jp305709y

  22. [22]

      S. Uzun, J. Ilavsky, G.W. Padua, Soft Matter 13(2017) 3053-3060. doi: 10.1039/C6SM02717B

  23. [23]

      H. M. Ye, L.T. Hong, Y. Gao, J. Xu, Chin. Chem. Lett. 28(2017) 888-892. doi: 10.1016/j.cclet.2016.12.032

  24. [24]

      H. Lee, K.H. Song, S.S. Im, et al., Nanosci. Nanotechnol. Lett. 8(2016) 168-172. doi: 10.1166/nnl.2016.2072

  25. [25]

      Y. Li, Q. Huang, T. Shi, L. An, J. Chem. Phys. 125(2006) 044902. doi: 10.1063/1.2213610

  26. [26]

      O. Glatter, O. Kratky, Small Angle X-ray Scattering, Academic Press, London, 1982.

  27. [27]

      K.C. Pratt, W.A. Wakeham, J. Chem. Soc. Faraday Trans. 73(1977) 997-1002. doi: 10.1039/f29777300997

  28. [28]

      P. Debye, A.M. Bueche, J. Appl. Phys. 20(1949) 518-525. doi: 10.1063/1.1698419

  29. [29]

      A.V. Semenyuk, D.I. Svergun, J. Appl. Crystallogr. 24(1991) 537-540. doi: 10.1107/S002188989100081X

  30. [30]

      D.I. Svergun, M.H.J. Koch, Rep. Prog. Phys. 66(2003) 1735-1782. doi: 10.1088/0034-4885/66/10/R05

  31. [31]

      Y. Li, Q. Huang, T. Shi, L. An, J. Phys. Chem. B 110(2006) 23502-23506. doi: 10.1021/jp0632790

  32. [32]

      S. Chodankar, V.K. Aswal, J. Kohlbrecher, R. Vavrin, A.G. Wagh, Phys. Rev. E 78(2008)031913. doi: 10.1103/PhysRevE.78.031913

  33. [33]

      Q.Z. Yu, Y. Li, M. Wang, H.Z. Chen, Chin. Chem. Lett. 19(2008) 223-226. doi: 10.1016/j.cclet.2007.12.005

• 

  Figure 1  SAXS intensity profiles (a) and the corresponding Debye-Bueche plots (b) of zein in 80% aqueous ethanol solutions. The profiles (c) and the corresponding pair distance distribution function (d) of PEO in 80% aqueous ethanol solutions. The profiles of zein-PEO mixed solutions (e) with fixed total concentration of 16 wt% at different fractions of zein, and the schematic plot for the structure change against the fraction of zein, aging time and total concentration (f). Lines in (a, b, c, e) are the best fitting to either a fractal zone or the original experimental points. Ellipsoid model is used to fit SAXS signal of zein in (a), and Debye model [28] is used to fit SAXS signal of PEO in (c). Experimental errors are less than the size of symbol so error bars are ignored.

  下载: 全尺寸图片 幻灯片
  

  Figure 2  SAXS intensity profiles (a) and Holtzer plots (b) for 120 mg/mL zein solutions at aging time of 2.5 h and 4 days.

  下载: 全尺寸图片 幻灯片
  

  Figure 3  Viscosity of zein in 80% (v/v) aqueous ethanol solutions at different aging time (a), and oscillatory time sweep at 1 Hz and 0.3% strain for 25 wt% zein solutions (b).

  下载: 全尺寸图片 幻灯片
  

  Figure 4  Morphologies of electrospun products captured by SEM at different concentrations, fractions of zein and aging time, and the total concentrations were labeled. Images a→b & a'→b', f(zein) from 0.3 to 0.9 from fresh solutions; images c→d & c'→d', aging time from fresh to 5 days with f(zein) of 1.0.

  下载: 全尺寸图片 幻灯片

  Table 1.  Structure and dynamic parameters derived from SAXS and rheology experiments for zein and PEO in aqueous ethanol solutions. The fraction of zein was fixed at 0.5 for the mixed solutions.

  下载: 导出CSV

  Table 2.  Structure parameters extracted from SAXS for fresh and aged zein solutions.

  下载: 导出CSV
•